What Percentage Of 10 Is 2
Treneri
May 13, 2025 · 4 min read
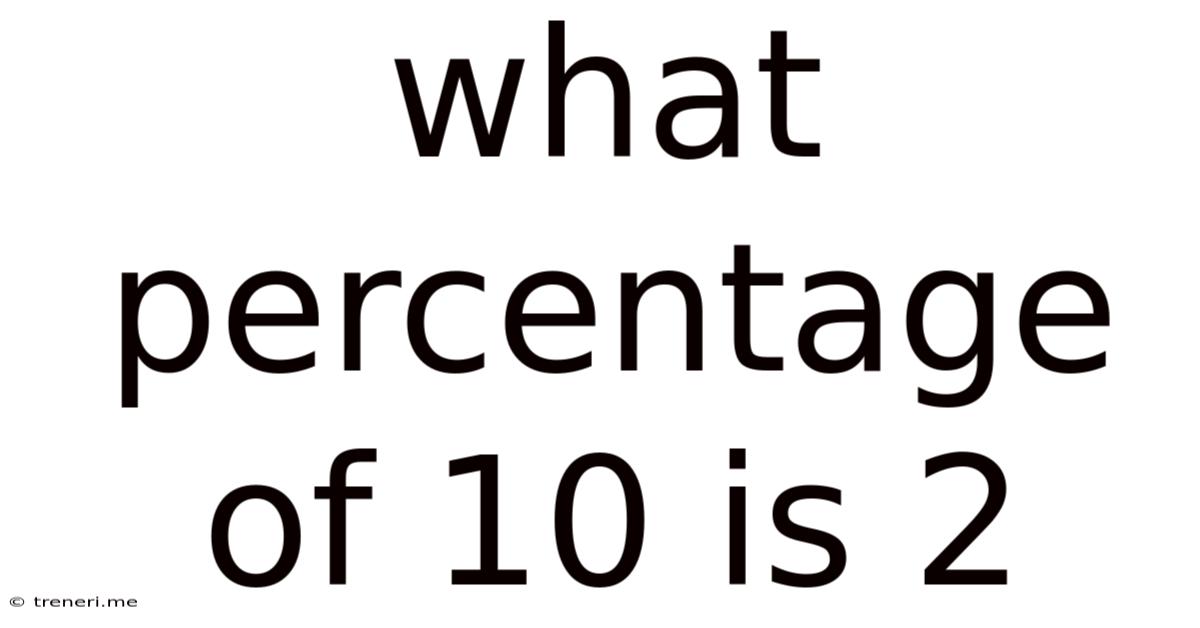
Table of Contents
What Percentage of 10 is 2? A Comprehensive Guide to Percentage Calculations
Understanding percentages is a fundamental skill applicable in numerous areas of life, from calculating discounts and taxes to comprehending statistical data and financial reports. This article delves into the seemingly simple question, "What percentage of 10 is 2?", but expands upon it to provide a comprehensive understanding of percentage calculations, offering various methods and practical applications.
Understanding Percentages: The Basics
A percentage represents a fraction of 100. The term "percent" literally means "out of one hundred." Therefore, 10% means 10 out of 100, which can be expressed as the fraction 10/100 or the decimal 0.1.
Key Concepts:
- Part: The specific amount you're considering (in our case, 2).
- Whole: The total amount you're referencing (in our case, 10).
- Percentage: The proportional representation of the part to the whole, expressed as a number followed by the "%" symbol.
Calculating "What Percentage of 10 is 2?"
There are several methods to determine what percentage of 10 is 2:
Method 1: The Formula Approach
The most straightforward method involves using the basic percentage formula:
(Part / Whole) * 100% = Percentage
Substituting our values:
(2 / 10) * 100% = 20%
Therefore, 2 is 20% of 10.
Method 2: Proportion Method
This method uses the concept of ratios and proportions. We can set up a proportion:
2 / 10 = x / 100
Where 'x' represents the unknown percentage. Solving for 'x':
10x = 200 x = 20
Therefore, x = 20%.
Method 3: Decimal Conversion
This method involves converting the fraction (Part/Whole) into a decimal and then multiplying by 100%:
2 / 10 = 0.2
0.2 * 100% = 20%
Practical Applications: Real-World Examples
Understanding percentage calculations is crucial in various real-life scenarios:
1. Discounts and Sales
Imagine a store offering a 20% discount on an item originally priced at $10. Using our knowledge, we know that 20% of $10 is $2, meaning the discount amount is $2, and the final price is $8.
2. Taxes and Fees
Calculating taxes involves similar principles. If a 5% sales tax is applied to a $20 purchase, we would calculate 5% of $20, which is $1, resulting in a total cost of $21.
3. Financial Analysis
Investors often use percentages to analyze financial data. For example, understanding the percentage change in a company's stock price or profit margin requires these calculations.
4. Statistical Analysis
Percentages are fundamental in interpreting and presenting statistical data. For example, expressing the percentage of people who voted for a particular candidate or the percentage of students who passed an exam.
5. Everyday Life
From calculating tips in restaurants to figuring out the percentage of ingredients in a recipe, percentage calculations are integrated into many aspects of daily life.
Expanding the Concept: Working with Different Values
While our example focused on "What percentage of 10 is 2?", the same principles apply to different values. Let's explore a few more examples:
Example 1: What percentage of 50 is 15?
Using the formula: (15 / 50) * 100% = 30%
Example 2: What percentage of 25 is 7?
Using the formula: (7 / 25) * 100% = 28%
Example 3: What percentage of 100 is 35?
Using the formula: (35 / 100) * 100% = 35% (Note: this is a straightforward example where the percentage is easily identifiable).
Dealing with More Complex Scenarios
While the basic formula remains the same, some scenarios might require an extra step or two:
Scenario 1: Finding the Whole when the Part and Percentage are Known:
For example: 20% of what number is 4?
Here, we rearrange the formula: (Part / Percentage) * 100 = Whole
(4 / 20) * 100 = 20
Therefore, 20% of 20 is 4.
Scenario 2: Finding the Part when the Whole and Percentage are Known:
For example: What is 30% of 50?
Here, we use the standard formula: (Percentage/100) * Whole = Part
(30/100) * 50 = 15
Therefore, 30% of 50 is 15.
Advanced Applications and Further Learning
The foundation provided here can be expanded into more advanced topics such as:
- Compound interest calculations: These involve percentages applied repeatedly over time.
- Statistical analysis: Understanding how percentages are used in hypothesis testing, confidence intervals, and regression analysis.
- Financial modeling: Developing complex financial models using percentage-based projections and scenarios.
Conclusion
The seemingly simple question, "What percentage of 10 is 2?", opens the door to a wider understanding of percentage calculations. This crucial skill, applicable across numerous disciplines and daily life situations, is essential for anyone seeking to improve their numerical literacy and analytical abilities. Mastering percentage calculations empowers you to interpret data more effectively, make informed decisions, and navigate the quantitative aspects of the world around you with greater confidence. By understanding the fundamental concepts, formulas, and practical applications outlined in this comprehensive guide, you can confidently tackle any percentage-related problem that comes your way.
Latest Posts
Latest Posts
-
411 Rounded To The Nearest Hundred
May 13, 2025
-
What Size Mirror For A 24 Inch Vanity
May 13, 2025
-
Does Two Quarts Equal A Gallon
May 13, 2025
-
How Many Oz Is 1 5 Pounds
May 13, 2025
-
Which Side Lengths Represent The Sides Of A Right Triangle
May 13, 2025
Related Post
Thank you for visiting our website which covers about What Percentage Of 10 Is 2 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.