What Percentage Of 5 Is 11
Treneri
May 09, 2025 · 4 min read
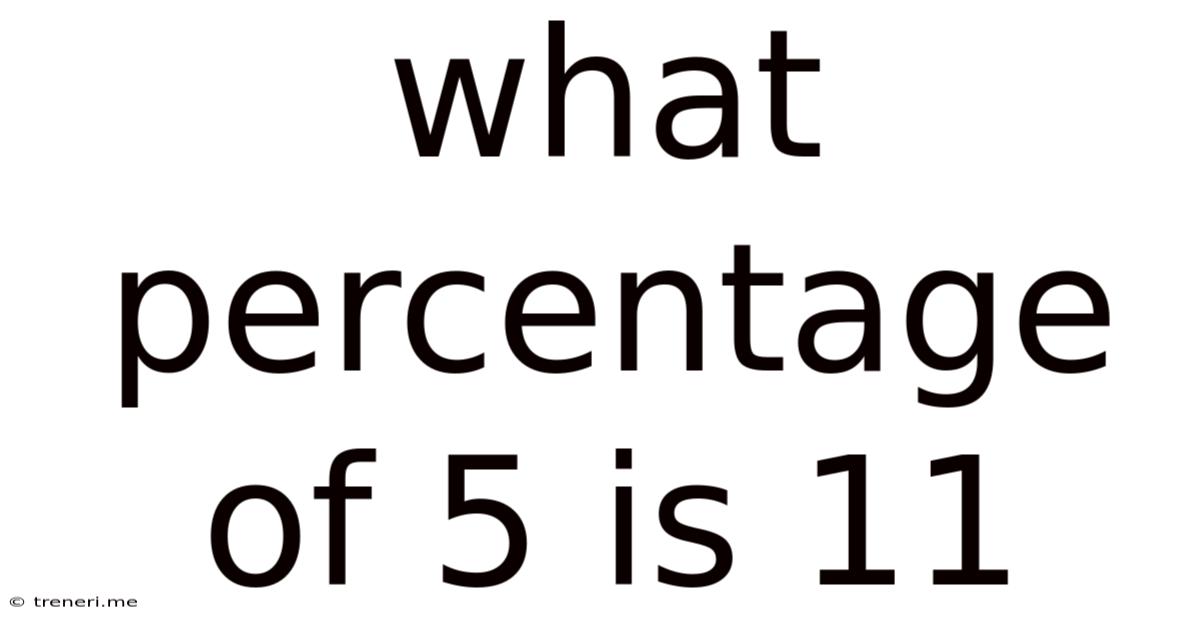
Table of Contents
What Percentage of 5 is 11? Understanding Percentages and Proportions
The question "What percentage of 5 is 11?" might seem straightforward at first glance, but it reveals a crucial concept in mathematics: understanding percentages as proportions. It's not a simple matter of directly dividing 11 by 5; the answer lies in understanding how percentages represent parts of a whole. This article will delve into the solution, exploring the underlying mathematical principles and providing practical examples to solidify your understanding.
Understanding Percentages
A percentage is a way of expressing a number as a fraction of 100. The symbol "%" represents "per cent," meaning "out of one hundred." For example, 50% means 50 out of 100, which is equivalent to the fraction 50/100 or the decimal 0.5. Understanding this fundamental concept is crucial for tackling percentage problems.
Setting up the Proportion
To find what percentage of 5 is 11, we need to set up a proportion. A proportion is a statement that two ratios are equal. In this case, our ratios will involve the percentage we're trying to find (let's call it 'x'), the number 11 (the part), and the number 5 (the whole).
We can write the proportion as:
x/100 = 11/5
This equation states that the ratio of 'x' to 100 (the percentage) is equal to the ratio of 11 to 5 (the part to the whole).
Solving the Proportion
To solve for 'x', we can use cross-multiplication:
5x = 11 * 100
5x = 1100
Now, divide both sides by 5:
x = 1100 / 5
x = 220
Therefore, 11 is 220% of 5.
Interpreting the Result: Percentages Greater Than 100%
Notice that our answer is greater than 100%. This is perfectly valid and simply means that the "part" (11) is larger than the "whole" (5). Percentages greater than 100% represent situations where a quantity exceeds the reference value. This often occurs in contexts like growth, increase, or comparisons where one value surpasses another.
Real-World Applications: Examples
Let's explore some real-world scenarios where understanding percentages greater than 100% is essential:
-
Investment Returns: If you invest $5 and your investment grows to $11, your return is 220% of your initial investment. This shows a substantial increase exceeding the original amount.
-
Sales Growth: If a company's sales increase from 5 million units to 11 million units, the sales growth is 220% of the original sales volume. This indicates significant expansion and market success.
-
Population Increase: If the population of a small town increases from 5,000 to 11,000, the population growth is 220% of the initial population. This points to a rapid growth rate.
-
Price Increases: Imagine an item costing $5 increases to $11. The price increase is 220% of the original price. This could reflect inflation, increased demand, or other market factors.
Alternative Methods for Calculating Percentages
While the proportion method is highly effective, let's examine other approaches to solving percentage problems:
Method 1: Using Decimal Conversion
First, find the fraction representing the relationship between the part and the whole: 11/5 = 2.2
Then, convert this fraction into a percentage by multiplying by 100: 2.2 * 100 = 220%
This method efficiently converts the fractional relationship directly into a percentage.
Method 2: Using a Calculator
Most calculators have a percentage function. You can simply input (11/5) * 100 to get the answer directly. This method is efficient for quick calculations.
Avoiding Common Mistakes
When working with percentages, several common errors can occur:
-
Incorrectly Identifying the Whole: Ensure you correctly identify the reference value (the "whole") before setting up the proportion or calculation.
-
Misinterpreting Percentages Greater Than 100%: Understand that percentages exceeding 100% indicate a value larger than the reference value.
-
Incorrect Calculation Order: Always follow the order of operations (PEMDAS/BODMAS) to avoid errors in calculations.
-
Rounding Errors: When rounding, maintain consistency and avoid premature rounding that can lead to inaccuracies in the final result.
Expanding Your Understanding: Percentage Change
This problem focuses on finding what percentage one number represents of another. A related concept is calculating percentage change. This involves comparing two values to determine the percentage increase or decrease between them. The formula for percentage change is:
[(New Value - Old Value) / Old Value] * 100
For instance, if a value increases from 5 to 11, the percentage change would be:
[(11 - 5) / 5] * 100 = 120%
This indicates a 120% increase, different from the 220% we calculated earlier because it focuses on the change rather than the final value as a proportion of the initial value.
Conclusion
The answer to "What percentage of 5 is 11?" is 220%. Understanding this involves grasping the concept of percentages as proportions, setting up and solving equations, and interpreting results accurately, particularly when dealing with percentages greater than 100%. This concept finds wide applicability in various real-world scenarios, from financial analysis to population studies and beyond. Mastering this mathematical principle enhances your ability to analyze data, interpret information, and make informed decisions. By understanding the underlying principles and practicing different methods, you can confidently tackle percentage problems and use this knowledge to solve various practical situations. Remember to always double-check your work and ensure you correctly identify the "whole" and "part" to avoid common errors. This will solidify your understanding of percentages and equip you with a valuable mathematical skill.
Latest Posts
Latest Posts
-
What Is 2 To The 20th Power
May 10, 2025
-
60 Days From December 10 2024
May 10, 2025
-
Greatest Common Factor Of 3 And 27
May 10, 2025
-
What Is The Greatest Common Factor Of 56 And 32
May 10, 2025
-
Which Quantity Of Heat Is Equal To 200 Joules
May 10, 2025
Related Post
Thank you for visiting our website which covers about What Percentage Of 5 Is 11 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.