Whats 25 Percent Off Of 60
Treneri
May 11, 2025 · 5 min read
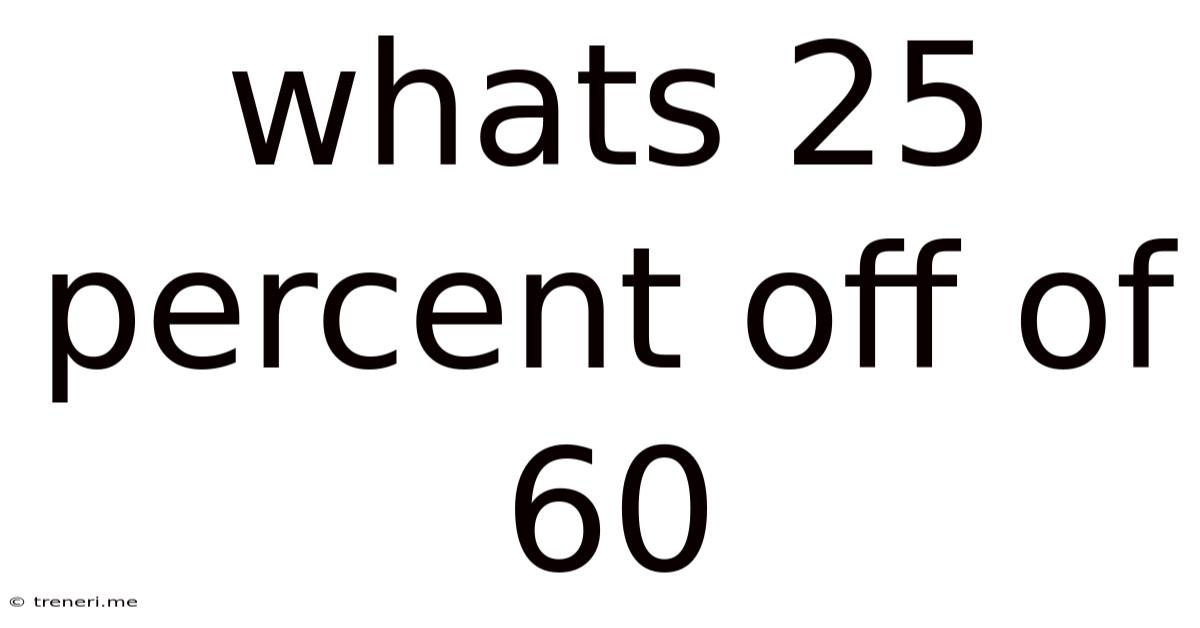
Table of Contents
What's 25 Percent Off of 60? A Comprehensive Guide to Percentage Calculations
Calculating discounts is a fundamental skill in everyday life, whether you're shopping for groceries, comparing prices, or negotiating deals. Understanding percentages allows you to quickly determine the final price after a discount, making informed purchasing decisions. This comprehensive guide will walk you through calculating 25% off of 60, explaining the methods involved and providing valuable insights into percentage calculations in general. We'll also explore different scenarios and applications, ensuring you're equipped to handle various percentage-related problems.
Understanding Percentages
Before diving into the calculation, let's solidify our understanding of percentages. A percentage is simply a fraction expressed as a part of 100. The symbol "%" represents "per hundred." For instance, 25% means 25 out of 100, or 25/100, which simplifies to 1/4.
This fundamental understanding is key to solving percentage problems. We can represent percentages as decimals or fractions, making the calculations more manageable depending on the context.
Method 1: Calculating 25% of 60
This method directly calculates 25% of 60, then subtracts this amount from the original price to find the discounted price.
Step 1: Convert the percentage to a decimal.
To convert 25% to a decimal, divide by 100:
25% ÷ 100 = 0.25
Step 2: Multiply the decimal by the original price.
Multiply the decimal (0.25) by the original price (60):
0.25 x 60 = 15
This means 25% of 60 is 15.
Step 3: Subtract the discount from the original price.
Subtract the calculated discount (15) from the original price (60):
60 - 15 = 45
Therefore, 25% off of 60 is $\boxed{45}$.
Method 2: Calculating the remaining percentage
This alternative method focuses on finding the remaining percentage after the discount and applying it to the original price.
Step 1: Determine the remaining percentage.
If we're taking 25% off, then the remaining percentage is 100% - 25% = 75%.
Step 2: Convert the remaining percentage to a decimal.
Convert 75% to a decimal by dividing by 100:
75% ÷ 100 = 0.75
Step 3: Multiply the decimal by the original price.
Multiply the decimal (0.75) by the original price (60):
0.75 x 60 = 45
This directly gives us the final discounted price: $\boxed{45}$.
Method 3: Using Fractions
This method leverages the fractional equivalent of 25%, which is 1/4.
Step 1: Express the percentage as a fraction.
25% is equivalent to 1/4.
Step 2: Multiply the fraction by the original price.
Multiply the fraction (1/4) by the original price (60):
(1/4) x 60 = 15
This gives us the discount amount, which is 15.
Step 3: Subtract the discount from the original price.
Subtract the discount (15) from the original price (60):
60 - 15 = 45
The final discounted price is again $\boxed{45}$.
Applying Percentage Calculations in Real-World Scenarios
Understanding percentage calculations extends far beyond simple discount problems. Here are some real-world applications:
1. Sales and Discounts:
Retail stores frequently offer discounts on merchandise. Being able to quickly calculate the final price after a discount is crucial for budget management. For example, a 30% discount on a $100 item can be easily calculated using the methods described above.
2. Taxes and Tips:
Calculating sales tax or adding a tip to a restaurant bill involves percentage calculations. For instance, a 6% sales tax on a $50 purchase requires multiplying 0.06 by 50 and adding the result to the original price.
3. Financial Calculations:
Percentage calculations are fundamental in finance, used to determine interest rates, returns on investments, and loan repayments. Understanding compound interest, for example, requires a strong grasp of percentage calculations.
4. Data Analysis:
Percentages are widely used in data analysis to represent proportions and trends. For example, representing market share, survey results, or changes in population requires accurate percentage calculations.
Advanced Percentage Problems and Strategies
While the problem of finding 25% off of 60 is relatively straightforward, understanding more complex percentage scenarios can be highly beneficial. Here are a few examples and strategies:
Finding the Original Price After a Discount
Let's say an item is now priced at $45 after a 25% discount. How do we find the original price?
This requires working backward. Since the $45 represents 75% (100% - 25%) of the original price, we can set up an equation:
0.75 * Original Price = 45
To find the original price, divide both sides by 0.75:
Original Price = 45 / 0.75 = 60
Therefore, the original price was $\boxed{60}$.
Calculating Percentage Increase or Decrease
Percentage change is another crucial aspect. Let's say a price increases from $50 to $60. What's the percentage increase?
Step 1: Calculate the difference:
60 - 50 = 10
Step 2: Divide the difference by the original value:
10 / 50 = 0.2
Step 3: Multiply by 100 to express as a percentage:
0.2 * 100 = 20%
Therefore, there's a $\boxed{20%}$ increase. A similar process can be used to calculate percentage decreases.
Dealing with Multiple Discounts
Sometimes you encounter multiple discounts applied sequentially. For example, a 20% discount followed by a 10% discount is not the same as a 30% discount. You must calculate the discounts step-by-step.
Let's say we have a $100 item with a 20% discount, followed by a 10% discount.
Step 1: First discount:
100 * 0.20 = 20 (discount) 100 - 20 = 80 (price after first discount)
Step 2: Second discount:
80 * 0.10 = 8 (discount) 80 - 8 = 72 (final price)
The final price is $\boxed{72}$, not $70 as a simple sum might suggest.
Conclusion
Calculating 25% off of 60 is a straightforward percentage problem with a solution of 45. However, mastering percentage calculations provides valuable skills for various situations, from shopping to financial planning and data analysis. By understanding the different methods and strategies presented in this guide, you can confidently tackle a wide range of percentage problems, empowering you to make informed decisions in everyday life. Remember to practice consistently, and you will quickly develop proficiency in solving percentage problems.
Latest Posts
Latest Posts
-
How Many Cups In 15 5 Oz
May 12, 2025
-
How Much Water Can A Swimming Pool Hold
May 12, 2025
-
What Is The Greatest Common Factor Of 32 And 36
May 12, 2025
-
1 8 2 3 As A Fraction
May 12, 2025
-
22 5 Out Of 25 As A Percentage
May 12, 2025
Related Post
Thank you for visiting our website which covers about Whats 25 Percent Off Of 60 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.