Whats 5 To The Power Of 3
Treneri
May 14, 2025 · 4 min read
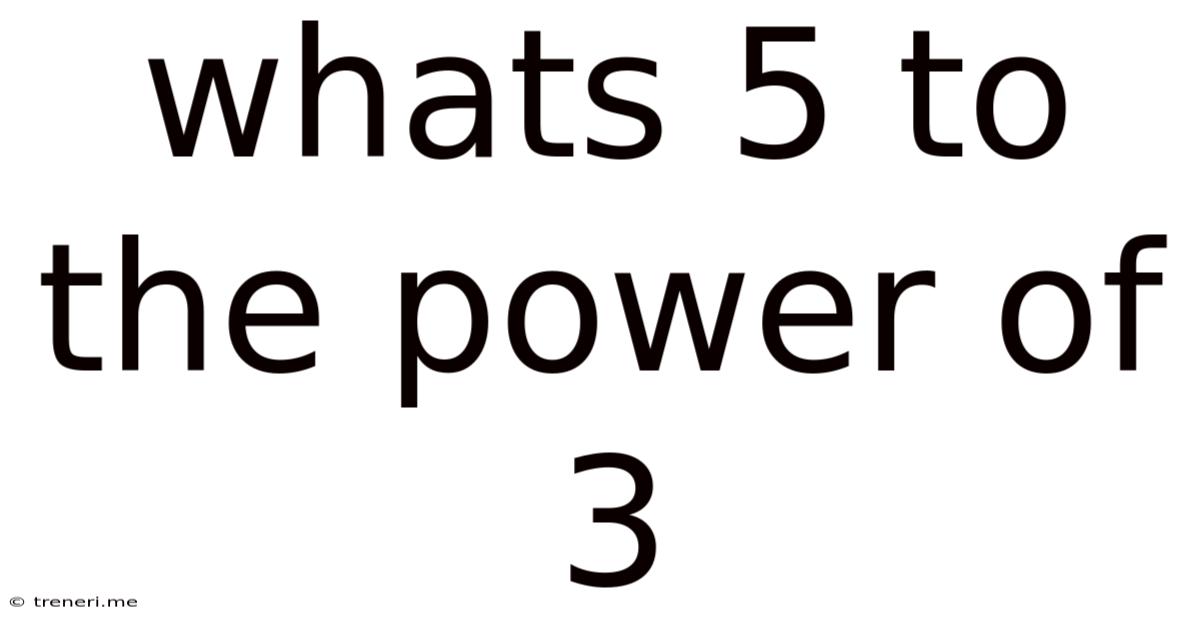
Table of Contents
What's 5 to the Power of 3? A Deep Dive into Exponents and Their Applications
The seemingly simple question, "What's 5 to the power of 3?" opens a door to a vast and fascinating world of mathematics, specifically the realm of exponents. While the answer itself is straightforward (125), understanding the concept and its applications unlocks a deeper appreciation for its significance across various fields. This article will delve into the meaning of exponents, explore the calculation of 5³, examine its relevance in different contexts, and uncover some surprising connections to other mathematical concepts.
Understanding Exponents: A Foundation in Mathematics
Exponents, also known as powers or indices, represent repeated multiplication. The expression 5³ (read as "5 to the power of 3" or "5 cubed") signifies 5 multiplied by itself three times: 5 x 5 x 5. The base number (5 in this case) is the number being multiplied, and the exponent (3) indicates how many times the base is multiplied by itself.
Key Terminology
Before we proceed, let's clarify some fundamental terminology:
- Base: The number being raised to a power (e.g., 5 in 5³).
- Exponent (or Power or Index): The number indicating how many times the base is multiplied by itself (e.g., 3 in 5³).
- Result (or Value): The outcome of the exponentiation (e.g., 125 in 5³).
Understanding these terms is crucial for grasping the concept of exponents and working with them effectively.
Calculating 5 to the Power of 3: A Step-by-Step Approach
Calculating 5³ is a simple process:
- Write out the expression: 5³
- Expand the expression: 5 x 5 x 5
- Perform the multiplication: 25 x 5 = 125
Therefore, 5 to the power of 3 equals 125.
While this calculation is straightforward for small numbers like 5³, the concept of exponents becomes incredibly powerful when dealing with larger numbers or variables.
The Significance of Exponents in Various Fields
The application of exponents extends far beyond simple mathematical calculations. They play a crucial role in numerous fields, including:
1. Science and Engineering
Exponents are fundamental in scientific notation, a way to represent very large or very small numbers concisely. For example, the speed of light is approximately 3 x 10⁸ meters per second, where 10⁸ represents 10 multiplied by itself eight times (100,000,000). Exponents are also essential in physics, chemistry, and engineering for describing exponential growth and decay, such as radioactive decay or population growth.
2. Finance and Economics
Compound interest calculations rely heavily on exponents. The formula for compound interest involves raising the principal amount to the power of the number of compounding periods. This allows for accurate prediction of future investment values. Exponential growth models are also used extensively in economic forecasting and analysis to understand trends in economic indicators.
3. Computer Science
Exponents are crucial in algorithms and data structures. Binary numbers, the foundation of computer systems, utilize powers of 2. The size of data structures, like arrays and trees, often involves exponential relationships, influencing algorithm efficiency and performance. Cryptographic algorithms also employ exponentiation for secure data encryption and decryption.
4. Statistics and Probability
Probability calculations often involve exponents. For instance, binomial probability distributions use exponents in their formulas to calculate the likelihood of a specific outcome in a series of independent trials. In statistical modeling, exponential functions are utilized to describe various phenomena, such as the distribution of certain random variables.
Beyond 5³: Exploring Higher Powers and Negative Exponents
While this article focuses on 5³, understanding the broader context of exponents is crucial. Let's explore some related concepts:
1. Higher Powers of 5
We can extend the concept to higher powers of 5:
- 5⁴ = 5 x 5 x 5 x 5 = 625
- 5⁵ = 5 x 5 x 5 x 5 x 5 = 3125
- and so on...
As the exponent increases, the result grows exponentially.
2. Negative Exponents
Negative exponents represent the reciprocal of the positive exponent. For example:
- 5⁻¹ = 1/5 = 0.2
- 5⁻² = 1/5² = 1/25 = 0.04
- 5⁻³ = 1/5³ = 1/125 = 0.008
Negative exponents result in values less than 1.
3. Fractional Exponents (Roots)
Fractional exponents represent roots. For example:
- 5^(1/2) = √5 (the square root of 5)
- 5^(1/3) = ³√5 (the cube root of 5)
Fractional exponents introduce a new dimension to the concept of exponentiation, connecting it to the extraction of roots.
The Power of Understanding: Conclusion
The seemingly simple calculation of 5³ unveils a rich tapestry of mathematical concepts and practical applications across various fields. Understanding exponents is fundamental to comprehending numerous scientific, financial, and computational phenomena. From exponential growth models to complex algorithms, exponents provide a powerful tool for describing and manipulating quantities in a concise and meaningful way. This exploration of 5³ serves not only as an answer to a specific question but as a gateway to a deeper appreciation of the power and elegance of mathematics. By grasping the fundamentals of exponents, we unlock a deeper understanding of the world around us, enabling us to solve complex problems and contribute to various fields of knowledge. The journey beyond 5³ is a continuous exploration, filled with fascinating discoveries and new applications of this fundamental mathematical concept.
Latest Posts
Latest Posts
-
1964 To 2023 How Many Years
May 14, 2025
-
How Long Is A 10 Mb Video
May 14, 2025
-
3995 Rounded To The Nearest Ten
May 14, 2025
-
2 1 10 As An Improper Fraction
May 14, 2025
-
Greatest Common Factor Of 63 And 72
May 14, 2025
Related Post
Thank you for visiting our website which covers about Whats 5 To The Power Of 3 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.