1 3 Divided By 2 5 In Fraction Form
Treneri
May 09, 2025 · 4 min read
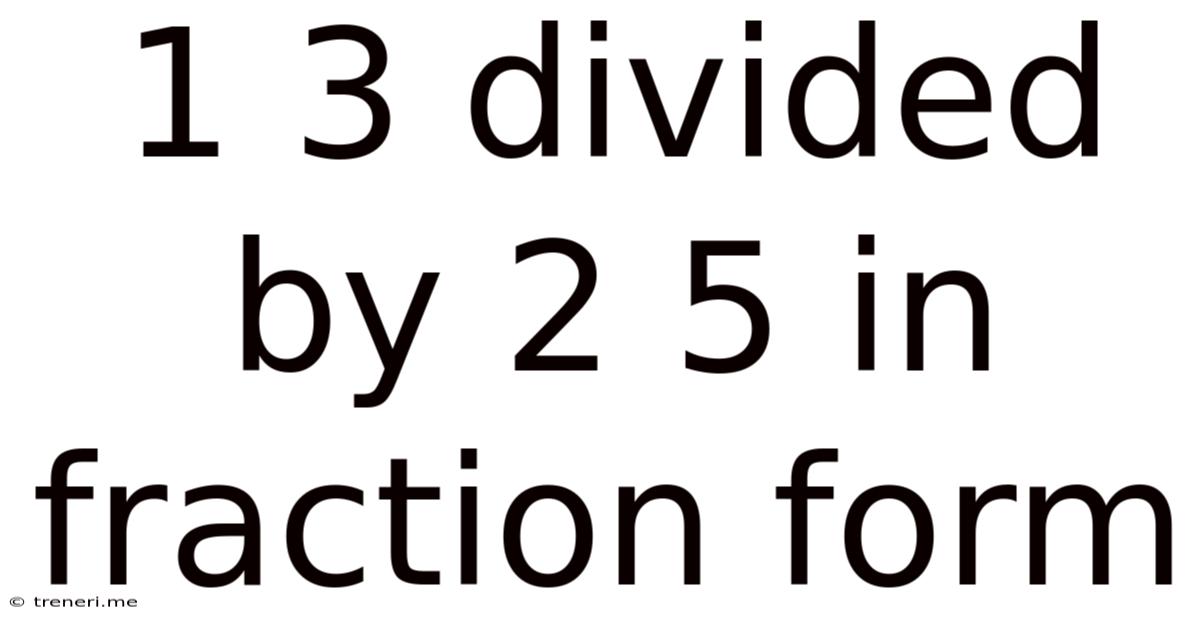
Table of Contents
1 3/5 Divided by 2 1/5 in Fraction Form: A Comprehensive Guide
This article will comprehensively guide you through solving the division problem: 1 3/5 divided by 2 1/5, focusing on the conversion to fractions and the step-by-step solution. We'll explore the underlying principles of fraction division and provide practical examples to solidify your understanding. By the end, you'll not only know the answer but also possess a robust understanding of the process, equipping you to tackle similar problems with confidence.
Understanding Fraction Division
Before tackling our specific problem, let's review the fundamentals of dividing fractions. The key concept is to invert the second fraction (the divisor) and multiply. This seemingly simple rule stems from the mathematical properties of reciprocals and division. Let's illustrate this:
a/b ÷ c/d = a/b × d/c
Where 'a', 'b', 'c', and 'd' represent numbers, and 'b' and 'c' are not zero (division by zero is undefined).
This principle remains true even when dealing with mixed numbers (numbers containing both whole and fractional parts), as we will see in our example.
Converting Mixed Numbers to Improper Fractions
Our problem, 1 3/5 ÷ 2 1/5, involves mixed numbers. Before applying the division rule for fractions, we must convert these mixed numbers into improper fractions. An improper fraction has a numerator (top number) larger than or equal to its denominator (bottom number).
Here's how to convert mixed numbers to improper fractions:
- Multiply the whole number by the denominator: For 1 3/5, this is 1 * 5 = 5.
- Add the numerator to the result: 5 + 3 = 8.
- Keep the same denominator: The denominator remains 5.
Therefore, 1 3/5 becomes 8/5.
Following the same steps for 2 1/5:
- Multiply the whole number by the denominator: 2 * 5 = 10.
- Add the numerator to the result: 10 + 1 = 11.
- Keep the same denominator: The denominator remains 5.
Therefore, 2 1/5 becomes 11/5.
Performing the Division: Step-by-Step Solution
Now that we've converted our mixed numbers to improper fractions, we can perform the division:
8/5 ÷ 11/5
Applying our rule of inverting and multiplying:
8/5 × 5/11
Notice that we've inverted 11/5 to become 5/11. Now we multiply the numerators together and the denominators together:
(8 × 5) / (5 × 11) = 40/55
Simplifying the Fraction
The fraction 40/55 is not in its simplest form. To simplify, we find the greatest common divisor (GCD) of the numerator (40) and the denominator (55). The GCD is the largest number that divides both 40 and 55 without leaving a remainder. In this case, the GCD is 5.
We divide both the numerator and the denominator by the GCD:
40 ÷ 5 = 8 55 ÷ 5 = 11
Therefore, the simplified fraction is 8/11.
The Complete Solution: A Concise Summary
To reiterate the entire process:
- Convert mixed numbers to improper fractions: 1 3/5 becomes 8/5, and 2 1/5 becomes 11/5.
- Invert the second fraction (divisor) and multiply: 8/5 ÷ 11/5 becomes 8/5 × 5/11.
- Multiply the numerators and denominators: (8 × 5) / (5 × 11) = 40/55.
- Simplify the fraction by finding the GCD and dividing: 40/55 simplifies to 8/11.
Therefore, the final answer to 1 3/5 divided by 2 1/5 is 8/11.
Practical Applications and Further Exploration
Understanding fraction division isn't just an academic exercise. It's a fundamental skill applicable to various real-world scenarios, including:
- Cooking and Baking: Scaling recipes up or down requires accurate fractional calculations.
- Sewing and Crafting: Precise measurements often involve fractions.
- Construction and Engineering: Accurate measurements and calculations are critical for building projects.
- Data Analysis: Many statistical calculations involve fractions.
Beyond this specific problem, you can use the principles outlined here to solve other fraction division problems. Practice is key to mastering this skill. Try working through various examples, gradually increasing the complexity of the fractions involved.
Troubleshooting Common Mistakes
Here are some common errors to watch out for when dividing fractions:
- Forgetting to invert the second fraction: This is the most common mistake. Always remember to invert (reciprocate) the fraction you're dividing by.
- Incorrectly converting mixed numbers to improper fractions: Double-check your calculations when converting mixed numbers. A small error here can significantly impact the final result.
- Not simplifying the final fraction: Always simplify your answer to its lowest terms. This ensures your answer is concise and accurate.
Conclusion
Mastering fraction division, including problems involving mixed numbers, is crucial for various mathematical and real-world applications. By understanding the steps involved – converting mixed numbers to improper fractions, inverting and multiplying, and simplifying the resulting fraction – you can confidently tackle any fraction division problem. Remember to practice regularly to solidify your understanding and build your problem-solving skills. This comprehensive guide provides a solid foundation to embark on your journey to becoming proficient in fraction arithmetic. Keep practicing, and you'll become increasingly comfortable and confident in working with fractions.
Latest Posts
Latest Posts
-
What Is The Gcf Of 39 And 65
May 11, 2025
-
Cuantos Pies Tiene Una Yarda De Cemento
May 11, 2025
-
How Many Month Is 120 Days
May 11, 2025
-
What Is 90 Days From June 6 2024
May 11, 2025
-
What Fractions Are Equal To 2 4
May 11, 2025
Related Post
Thank you for visiting our website which covers about 1 3 Divided By 2 5 In Fraction Form . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.