1 5 Divided By 1 3
Treneri
May 10, 2025 · 5 min read
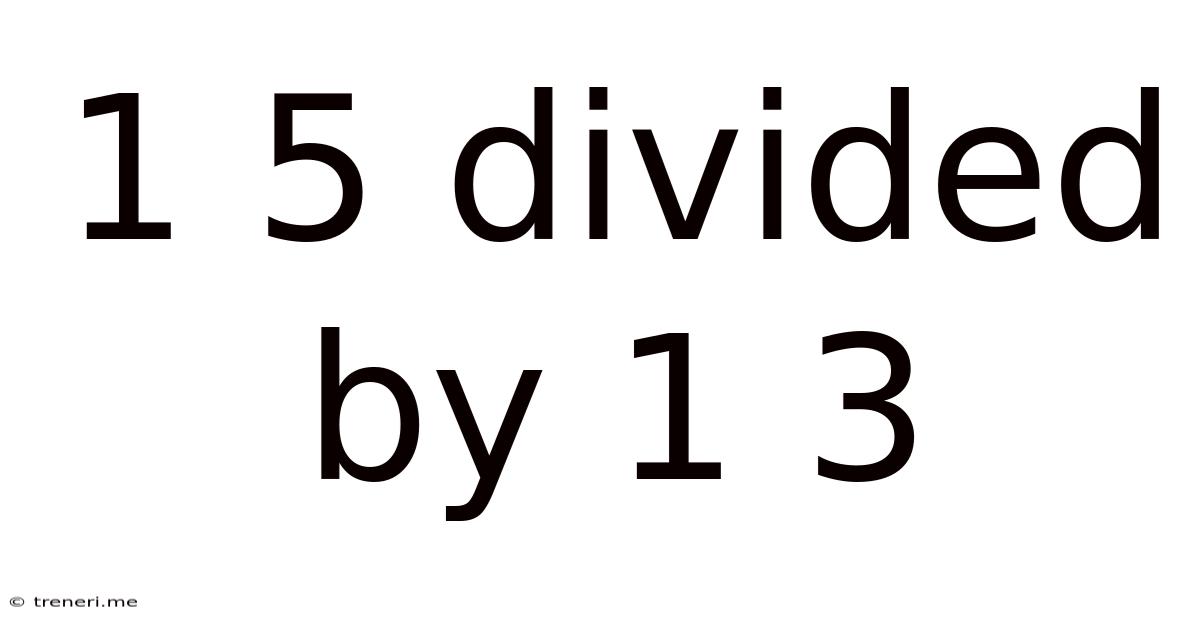
Table of Contents
1.5 Divided by 1.3: A Deep Dive into Decimal Division
This article will explore the seemingly simple calculation of 1.5 divided by 1.3, delving far beyond the immediate answer. We'll examine various methods for solving this problem, discuss the underlying mathematical concepts, and explore real-world applications where such calculations are crucial. By the end, you'll not only know the answer but also understand the 'why' behind the process, solidifying your understanding of decimal division.
Understanding Decimal Division
Before diving into the specific calculation, let's refresh our understanding of decimal division. Decimal division involves dividing numbers that contain a decimal point. It's a fundamental arithmetic operation with broad applicability in various fields, from basic accounting to complex engineering calculations.
The core concept remains the same as whole-number division: we're determining how many times one number (the divisor) goes into another number (the dividend). The key difference lies in managing the decimal point.
There are several methods for performing decimal division, each with its own strengths and weaknesses:
1. Long Division
Long division is a traditional method that provides a step-by-step breakdown of the division process. While it might seem laborious, it's a robust method that fosters a deep understanding of the underlying principles.
To solve 1.5 ÷ 1.3 using long division, we can follow these steps:
-
Eliminate the decimal points: Multiply both the dividend and the divisor by a power of 10 to make them whole numbers. In this case, multiplying both by 10 gives us 15 ÷ 13.
-
Perform long division:
1.1538... 13 | 15.0000 -13 20 -13 70 -65 50 -39 110 -104 6 -
Interpret the result: The result is approximately 1.1538. The "..." indicates that the decimal continues infinitely, as 15/13 is a rational number with a non-terminating, repeating decimal representation.
2. Using a Calculator
The simplest method, especially for complex decimals, is using a calculator. Modern calculators are designed to handle decimal division efficiently. Simply input 1.5 ÷ 1.3 and the calculator will provide the answer, usually with a certain degree of accuracy determined by the calculator's capacity.
3. Converting to Fractions
Another effective method involves converting the decimals into fractions. This approach can be particularly useful for understanding the underlying mathematical relationships.
-
Convert decimals to fractions: 1.5 can be expressed as 3/2, and 1.3 as 13/10.
-
Divide the fractions: Dividing fractions involves inverting the second fraction and multiplying: (3/2) ÷ (13/10) = (3/2) * (10/13) = 30/26
-
Simplify the fraction: 30/26 simplifies to 15/13.
-
Convert back to decimal: Performing the long division of 15/13, as shown above, yields the decimal approximation of 1.1538...
Significance of the Result and its Applications
The result of 1.5 ÷ 1.3, approximately 1.1538, holds significance in various contexts. Understanding its application highlights the practical relevance of decimal division.
Real-World Applications:
-
Financial Calculations: Dividing expenses by income to calculate expense ratios or determining the number of units that can be purchased with a given budget.
-
Engineering and Physics: Calculating ratios, proportions, and scaling factors in various design and scientific calculations.
-
Chemistry and Science: Determining concentrations, molar ratios, or converting units in chemical calculations.
-
Data Analysis: Normalizing data, calculating percentages, or finding averages.
-
Construction and Architecture: Calculating material quantities, dimensions, or determining proportions in building designs.
-
Everyday tasks: Dividing a recipe to serve fewer people or determining the unit cost of an item.
Exploring the Remainder and Rounding
The long division reveals that 15/13 doesn't result in a terminating decimal. This is due to the nature of the fraction, specifically the fact that the denominator (13) contains prime factors other than 2 and 5. The remainder in the long division process signifies that the division is not exact. In practical applications, we often need to round the result to a suitable degree of precision.
The choice of rounding depends on the context:
- Rounding to one decimal place: 1.2
- Rounding to two decimal places: 1.15
- Rounding to three decimal places: 1.154
- Rounding to four decimal places: 1.1538
The level of accuracy required dictates the appropriate rounding method. Overly precise rounding isn't always necessary and can even be misleading. Consider the context and the purpose of your calculation when choosing a rounding method.
Further Exploration: Recurring Decimals and Rational Numbers
The result of 1.5 ÷ 1.3, 1.1538..., exemplifies a recurring decimal. Recurring decimals are decimal representations of rational numbers (fractions) that have a repeating pattern of digits after the decimal point. In this case, the repeating pattern isn't immediately obvious because we stopped the long division at a certain point. Continued division would reveal the repeating pattern.
Understanding recurring decimals is crucial for working with fractions and performing accurate calculations. They demonstrate the limitations of representing some rational numbers precisely using decimals.
Conclusion: Mastering Decimal Division
This comprehensive exploration of 1.5 divided by 1.3 highlights the importance of understanding the underlying principles of decimal division. Whether using long division, a calculator, or converting to fractions, selecting the most efficient method depends on the complexity of the calculation and the level of accuracy required. Understanding the concept of recurring decimals and the appropriate rounding techniques is equally crucial for applying this fundamental mathematical operation effectively in various real-world scenarios. The seemingly simple calculation of 1.5 ÷ 1.3 opens doors to a deeper understanding of numbers, fractions, and their diverse applications. Remember that the core principles remain consistent, regardless of the specific numbers involved. With practice and a solid understanding of the underlying concepts, you can confidently tackle any decimal division problem you encounter.
Latest Posts
Latest Posts
-
What Is A 6 12 Roof Pitch
May 10, 2025
-
How To Find The Missing Base Of A Trapezoid
May 10, 2025
-
24 X 24 How Many Square Feet
May 10, 2025
-
What Percentage Is 60 Out Of 70
May 10, 2025
-
Cuantos Dias Faltan Para El Sabado
May 10, 2025
Related Post
Thank you for visiting our website which covers about 1 5 Divided By 1 3 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.