32 9 As A Mixed Number
Treneri
May 11, 2025 · 5 min read
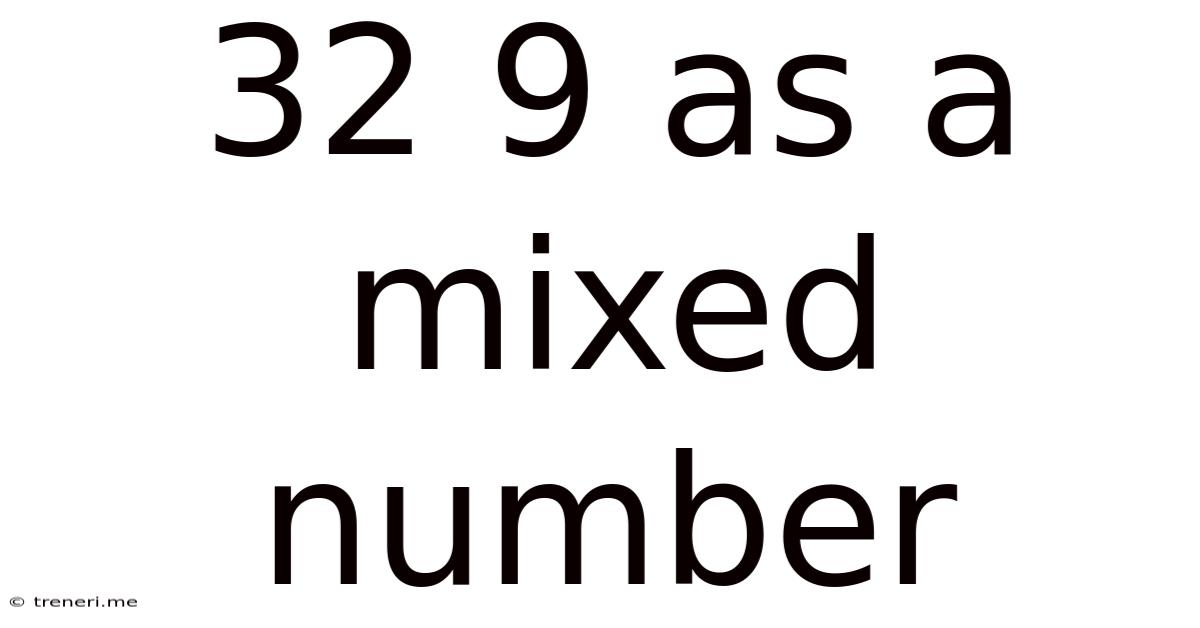
Table of Contents
32/9 as a Mixed Number: A Comprehensive Guide
Converting improper fractions, like 32/9, into mixed numbers is a fundamental skill in arithmetic. This comprehensive guide will not only show you how to convert 32/9 into a mixed number but will also delve into the underlying concepts, provide alternative methods, and offer practical applications to solidify your understanding. We'll explore various approaches, ensuring you grasp the process fully and can apply it to other improper fractions with confidence.
Understanding Improper Fractions and Mixed Numbers
Before we dive into the conversion of 32/9, let's clarify the definitions:
-
Improper Fraction: An improper fraction is a fraction where the numerator (the top number) is greater than or equal to the denominator (the bottom number). Examples include 7/4, 11/5, and, of course, 32/9.
-
Mixed Number: A mixed number combines a whole number and a proper fraction. A proper fraction has a numerator smaller than its denominator. Examples include 1 ¾, 2 ⅔, and 3 ⅛.
The core concept behind converting an improper fraction to a mixed number is to determine how many times the denominator goes into the numerator, representing the whole number part, and then expressing the remainder as a fraction.
Method 1: Long Division
The most common and perhaps easiest method to convert 32/9 into a mixed number involves long division.
Steps:
-
Divide the numerator by the denominator: Divide 32 by 9.
-
Determine the whole number: The quotient (the result of the division) represents the whole number part of the mixed number. 32 divided by 9 is 3 with a remainder.
-
Find the remainder: The remainder is the leftover amount after the division. In this case, the remainder is 5 (9 x 3 = 27; 32 - 27 = 5).
-
Express the remainder as a fraction: The remainder becomes the numerator of the fraction, and the original denominator (9) remains the denominator. This gives us the fraction 5/9.
-
Combine the whole number and the fraction: Combine the whole number (3) and the fraction (5/9) to form the mixed number: 3 5/9.
Therefore, 32/9 as a mixed number is 3 5/9.
Method 2: Repeated Subtraction
This method is excellent for visualizing the process. It involves repeatedly subtracting the denominator from the numerator until the result is less than the denominator.
Steps:
-
Repeatedly subtract the denominator: Subtract 9 from 32 repeatedly:
- 32 - 9 = 23
- 23 - 9 = 14
- 14 - 9 = 5
-
Count the number of subtractions: We subtracted 9 three times. This is our whole number.
-
The final result is the remainder: The final result of the subtraction (5) is the remainder, which becomes the numerator of the fraction.
-
Form the mixed number: The whole number (3) and the remainder (5) over the original denominator (9) give us the mixed number: 3 5/9.
This method offers a different perspective on the conversion, strengthening your conceptual understanding.
Method 3: Using Multiplication and Subtraction (A less common but useful method)
This method utilizes the relationship between the improper fraction and its equivalent mixed number.
Steps:
-
Find the closest multiple of the denominator to the numerator: The closest multiple of 9 to 32 is 27 (9 x 3 = 27).
-
Subtract to find the remainder: Subtract this multiple from the numerator: 32 - 27 = 5.
-
Form the mixed number: The number of times we multiplied the denominator (3) becomes the whole number. The remainder (5) becomes the numerator of the fraction, and the denominator remains 9. This gives us: 3 5/9.
While less intuitive than the previous methods, this approach can be helpful in mentally estimating the mixed number equivalent.
Practical Applications and Real-World Examples
Understanding the conversion of improper fractions to mixed numbers is crucial in various real-world scenarios:
-
Measurement: Imagine you have 32 inches of ribbon, and you need to cut it into pieces that are 9 inches long. Converting 32/9 to 3 5/9 tells you that you can make three complete pieces, with 5/9 of an inch remaining.
-
Baking: Recipes often use fractions. If a recipe calls for 32/9 cups of flour, understanding that this is equivalent to 3 5/9 cups helps you measure accurately.
-
Sharing: If you have 32 cookies to share equally among 9 people, converting 32/9 to 3 5/9 tells you each person gets 3 whole cookies, and there are 5 cookies left to share.
-
Construction and Engineering: Precise measurements are critical in construction and engineering, and the ability to convert between improper fractions and mixed numbers is essential for accuracy.
Extending Your Understanding: Working with Larger Numbers
The principles outlined above apply to all improper fractions. Let's consider a larger example to further solidify your understanding. Convert 157/12 into a mixed number:
- Long Division: 157 ÷ 12 = 13 with a remainder of 1.
- Mixed Number: This gives us the mixed number 13 1/12.
Remember, the process remains the same: divide the numerator by the denominator, use the quotient as the whole number, and the remainder as the numerator of the fraction, keeping the original denominator.
Simplifying Mixed Numbers
Sometimes, the fractional part of a mixed number can be simplified. For example, if we had obtained a mixed number like 2 6/12, we can simplify the fraction 6/12 to ½, resulting in the simplified mixed number 2 ½. Always check your fraction to see if it can be simplified to its lowest terms.
Conclusion
Converting an improper fraction like 32/9 into its mixed number equivalent, 3 5/9, is a fundamental mathematical skill with wide-ranging practical applications. By mastering the various methods outlined in this guide—long division, repeated subtraction, and the multiplication/subtraction method—you will develop a strong understanding of fractions and their representation in different forms. Remember to practice regularly to reinforce your learning and confidently tackle more complex fraction conversions in various contexts. Understanding the intricacies of fraction manipulation will prove invaluable in various fields and everyday situations.
Latest Posts
Latest Posts
-
Gcf Of 15 12 And 10
May 13, 2025
-
Z Score For 93 Confidence Interval
May 13, 2025
-
What Is Equivalent To 6 10
May 13, 2025
-
How Much Is 1 8 1 8
May 13, 2025
-
14 Out Of 16 As A Grade
May 13, 2025
Related Post
Thank you for visiting our website which covers about 32 9 As A Mixed Number . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.