Gcf Of 15 12 And 10
Treneri
May 13, 2025 · 5 min read
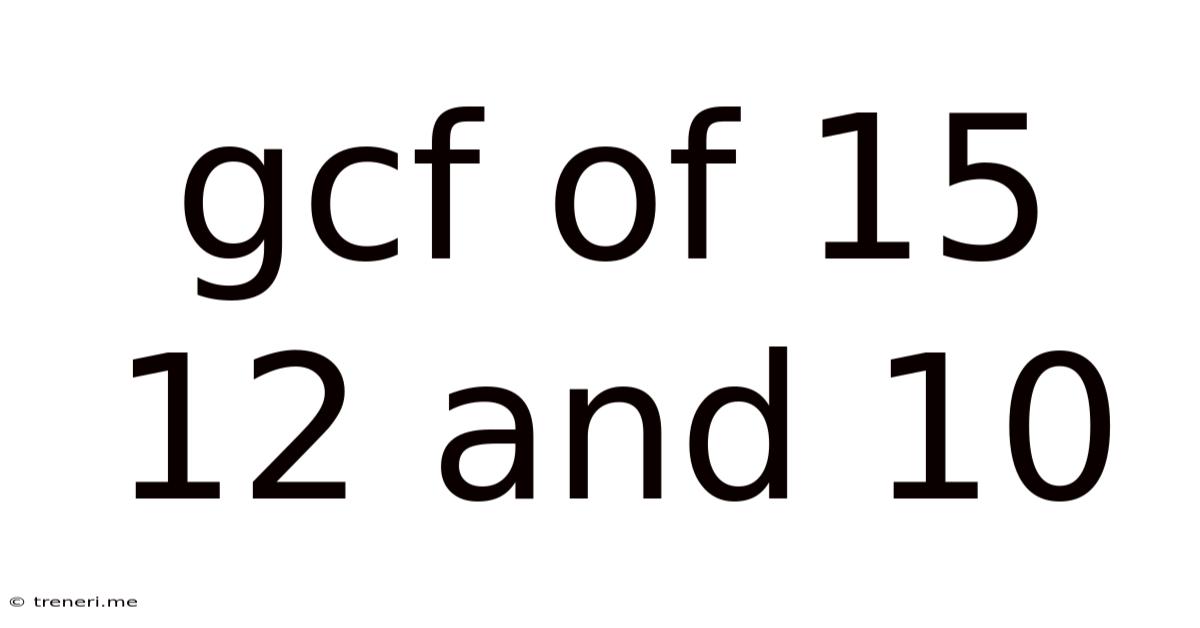
Table of Contents
Finding the Greatest Common Factor (GCF) of 15, 12, and 10: A Comprehensive Guide
Finding the greatest common factor (GCF), also known as the greatest common divisor (GCD), of a set of numbers is a fundamental concept in mathematics with applications across various fields, from simplifying fractions to solving complex algebraic equations. This article delves deep into the process of determining the GCF of 15, 12, and 10, exploring different methods and illustrating their practical applications. We'll also cover related concepts and provide examples to solidify your understanding.
Understanding the Greatest Common Factor (GCF)
The GCF of two or more numbers is the largest number that divides evenly into all of them without leaving a remainder. It's the largest factor common to all the numbers in the set. For example, the GCF of 12 and 18 is 6 because 6 is the largest number that divides both 12 and 18 without leaving a remainder.
This concept is crucial in various mathematical operations, including:
- Simplifying fractions: Finding the GCF helps reduce fractions to their simplest form.
- Solving algebraic equations: GCF plays a role in factoring polynomials.
- Measurement and Geometry: Calculating GCF is useful in problems involving area, volume, and other geometric measurements.
Methods for Finding the GCF of 15, 12, and 10
Several methods can be used to find the GCF of 15, 12, and 10. We'll explore the most common ones:
1. Listing Factors Method
This method involves listing all the factors of each number and identifying the largest factor common to all three.
- Factors of 15: 1, 3, 5, 15
- Factors of 12: 1, 2, 3, 4, 6, 12
- Factors of 10: 1, 2, 5, 10
Comparing the lists, we see that the common factors are only 1. Therefore, the GCF of 15, 12, and 10 is 1.
2. Prime Factorization Method
This method involves expressing each number as a product of its prime factors. The GCF is then found by multiplying the common prime factors raised to the lowest power.
- Prime factorization of 15: 3 x 5
- Prime factorization of 12: 2 x 2 x 3 = 2² x 3
- Prime factorization of 10: 2 x 5
Comparing the prime factorizations, we see that there are no common prime factors among all three numbers except for the implicit factor of 1, indicating the GCF is 1.
3. Euclidean Algorithm
The Euclidean algorithm is a highly efficient method for finding the GCF of two numbers. While it's primarily designed for two numbers, we can extend it to find the GCF of multiple numbers by finding the GCF of the first two numbers, and then finding the GCF of the result and the third number, and so on.
Let's apply it to 15, 12, and 10:
-
Find the GCF of 15 and 12:
- 15 = 1 x 12 + 3
- 12 = 4 x 3 + 0 The GCF of 15 and 12 is 3.
-
Find the GCF of 3 (the result from step 1) and 10:
- 10 = 3 x 3 + 1
- 3 = 3 x 1 + 0 The GCF of 3 and 10 is 1.
Therefore, the GCF of 15, 12, and 10 is 1.
Applications of Finding the GCF
The ability to find the GCF has numerous practical applications in various areas:
1. Simplifying Fractions
Consider the fraction 12/15. To simplify this fraction, we need to find the GCF of 12 and 15, which is 3. Dividing both the numerator and the denominator by 3, we get the simplified fraction 4/5. Similarly, consider 10/15; the GCF is 5, resulting in a simplified fraction of 2/3. Finally, 12/10; the GCF is 2, resulting in 6/5.
2. Solving Algebraic Equations
GCF is instrumental in factoring polynomials. Consider the expression 15x² + 12x + 10x. The GCF of 15, 12, and 10 is 1, and the GCF of the x terms is x. Therefore, the factored form of the expression is x(15x + 12 + 10).
3. Geometry and Measurement
Suppose you have three rectangular pieces of land with dimensions 15m x 12m, 12m x 10m, and 15m x 10m. If you want to divide each piece into identical square plots, the side length of the square plots would be the GCF of 15, 12, and 10, which is 1 meter. This ensures that each piece of land is divided into squares of the same size without any leftover space.
Understanding the Concept of Relatively Prime Numbers
When the GCF of two or more numbers is 1, these numbers are called relatively prime or coprime. In our case, 15, 12, and 10 are relatively prime to each other because their GCF is 1. This means they do not share any common factors other than 1.
Advanced Techniques and Considerations
For larger numbers or a greater number of numbers, the prime factorization method might become cumbersome. In such scenarios, advanced techniques like the Euclidean algorithm become particularly useful due to their efficiency. Furthermore, computer programs and calculators can easily compute the GCF of very large numbers.
Conclusion
Finding the GCF is a fundamental mathematical skill with broad applications. Whether you're simplifying fractions, factoring polynomials, or solving geometric problems, understanding and applying the various methods for calculating the GCF is crucial. The GCF of 15, 12, and 10, as we've demonstrated through multiple methods, is 1, highlighting the concept of relatively prime numbers. Mastering this concept provides a solid foundation for more advanced mathematical explorations. Remember to choose the method best suited to the problem at hand, keeping efficiency and accuracy in mind.
Latest Posts
Latest Posts
-
What Is The Greatest Common Factor Of 28 And 14
May 13, 2025
-
1 4 As An Improper Fraction
May 13, 2025
-
20 Cloves Of Garlic To Tbsp
May 13, 2025
-
3 Garlic Cloves Minced To Tbsp
May 13, 2025
-
15 Is 6 Percent Of What Number
May 13, 2025
Related Post
Thank you for visiting our website which covers about Gcf Of 15 12 And 10 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.