8 Is 40 Percent Of What Number
Treneri
May 13, 2025 · 4 min read
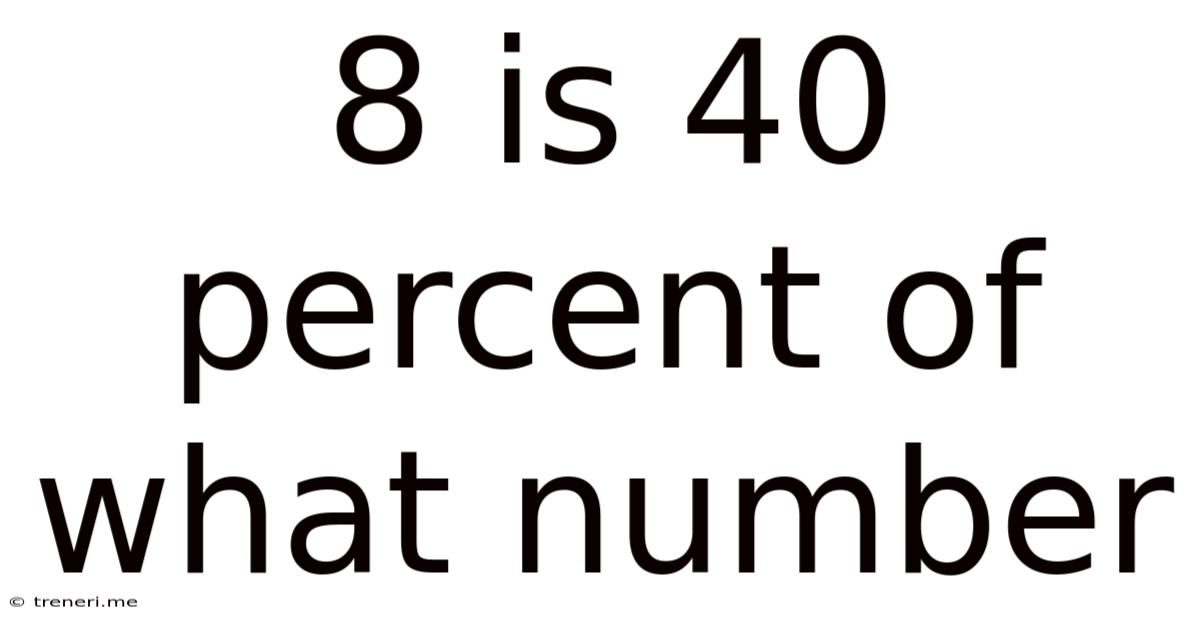
Table of Contents
8 is 40 Percent of What Number? A Comprehensive Guide to Percentage Calculations
Understanding percentages is a fundamental skill in many areas of life, from calculating discounts and taxes to analyzing data and understanding financial reports. This article delves into the question, "8 is 40 percent of what number?", providing a step-by-step explanation of how to solve this type of problem, exploring different methods, and offering practical applications and real-world examples. We'll also cover related concepts to build a strong foundation in percentage calculations.
Understanding Percentages
Before we tackle the specific problem, let's refresh our understanding of percentages. A percentage is a fraction or ratio expressed as a number out of 100. The symbol "%" denotes percentage. For example, 50% means 50 out of 100, which is equivalent to 50/100 or 1/2 (one-half).
Key Concepts:
- Percentage: The portion or fraction of a whole represented as a number out of 100.
- Part: The value that represents the percentage of a whole.
- Whole: The total value or the base amount.
Solving "8 is 40 Percent of What Number?"
There are several ways to solve this problem. Let's explore the most common methods:
Method 1: Using the Proportion Method
This method is based on setting up a proportion, which is an equation stating that two ratios are equal. We can represent the problem as:
8 / x = 40 / 100
Where:
- 8 represents the part.
- x represents the whole (the number we want to find).
- 40/100 represents 40% as a fraction.
To solve for x, we can cross-multiply:
8 * 100 = 40 * x
800 = 40x
Now, divide both sides by 40:
x = 800 / 40
x = 20
Therefore, 8 is 40% of 20.
Method 2: Using the Decimal Method
This method involves converting the percentage to a decimal and setting up an equation. First, convert 40% to a decimal by dividing by 100:
40% = 40 / 100 = 0.40
Now, we can set up the equation:
8 = 0.40 * x
To solve for x, divide both sides by 0.40:
x = 8 / 0.40
x = 20
Again, we find that 8 is 40% of 20.
Method 3: Using the Formula
A more generalized formula for percentage problems is:
Part = Percentage × Whole
In our problem:
- Part = 8
- Percentage = 40% = 0.40
- Whole = x (what we need to find)
Substituting these values into the formula:
8 = 0.40 * x
Solving for x (as shown in Method 2):
x = 8 / 0.40 = 20
Practical Applications and Real-World Examples
Understanding percentage calculations is crucial in various real-world scenarios. Here are a few examples:
-
Sales and Discounts: A store offers a 40% discount on an item, and the discount amount is $8. Using the methods above, we can determine that the original price of the item was $20.
-
Financial Analysis: If a company's profit is $8 million, representing 40% of its revenue, we can calculate its total revenue as $20 million.
-
Test Scores: If a student scored 8 points out of a possible 20 points, and this represents 40% of the total points, then this demonstrates a clear understanding of the test material.
-
Surveys and Statistics: If 8 out of 20 respondents (40%) chose a specific option in a survey, it helps in understanding the preference distribution.
-
Commission Calculations: If a salesperson earns a 40% commission and their commission amount is $800, their total sales would be $2000.
Expanding Your Understanding: Related Percentage Calculations
Mastering percentage calculations involves understanding related concepts and variations of problems. Here are some extensions to build your skills:
Finding the Percentage:
Let's say you have the part (8) and the whole (20). To find the percentage, use this formula:
Percentage = (Part / Whole) × 100
Percentage = (8 / 20) × 100 = 40%
Finding the Part:
If you know the percentage (40%) and the whole (20), you can find the part:
Part = Percentage × Whole
Part = 40% × 20 = 8
Finding the Percentage Increase or Decrease:
These calculations are essential for understanding changes over time or comparing values. The formula for percentage increase is:
Percentage Increase = [(New Value - Old Value) / Old Value] × 100
Similarly, for percentage decrease:
Percentage Decrease = [(Old Value - New Value) / Old Value] × 100
Conclusion
Solving "8 is 40 percent of what number?" is straightforward using various methods. The ability to perform percentage calculations accurately is a valuable skill applicable to many aspects of daily life and professional endeavors. By understanding the fundamental concepts and exploring different approaches, you can confidently tackle similar problems and build a solid foundation in quantitative reasoning. Remember to practice regularly to solidify your understanding and improve your proficiency in percentage calculations. This will allow you to confidently approach complex problems involving percentages, discounts, interest rates, and more, enabling you to make informed decisions and interpret data effectively.
Latest Posts
Latest Posts
-
How Many Days Is A 100 Hours
May 13, 2025
-
What Is The Gcf Of 15 And 6
May 13, 2025
-
The Triangular Prism Has A Volume Of 120
May 13, 2025
-
12 5 Is 8 Of What Number
May 13, 2025
-
How Many Minutes In 13 Years
May 13, 2025
Related Post
Thank you for visiting our website which covers about 8 Is 40 Percent Of What Number . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.