98 Divided By 13 With Remainder
Treneri
May 12, 2025 · 5 min read
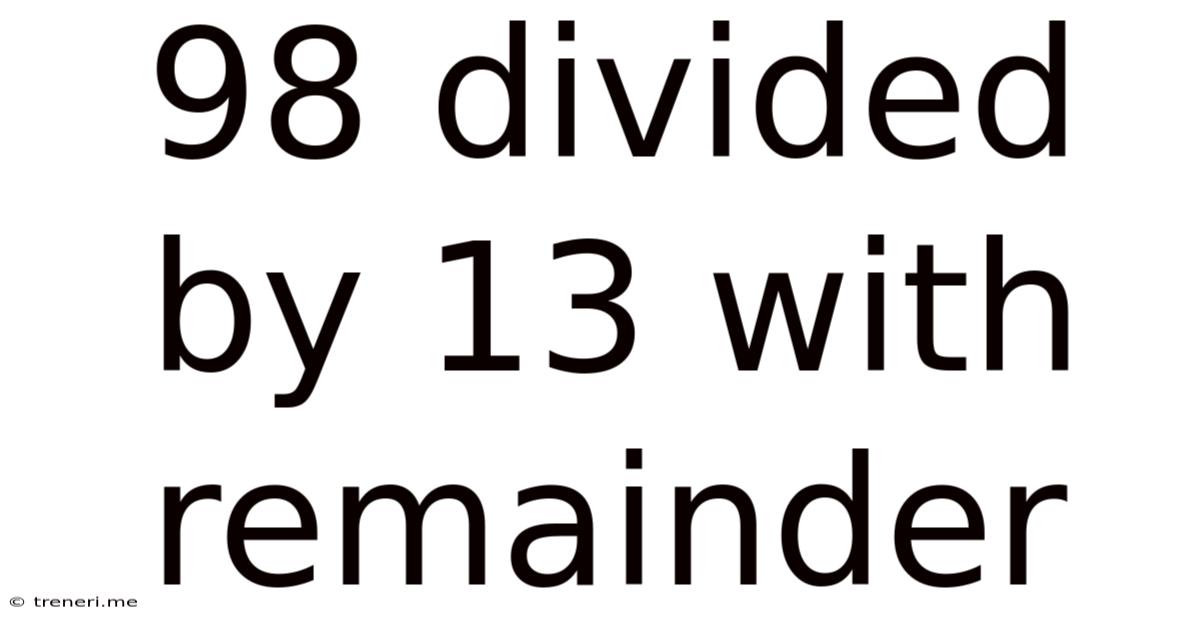
Table of Contents
98 Divided by 13: A Deep Dive into Division with Remainders
This seemingly simple arithmetic problem, 98 divided by 13, offers a fantastic opportunity to explore fundamental mathematical concepts and delve into the nuances of division, particularly when dealing with remainders. While a calculator can quickly provide the answer, understanding the process behind the solution is crucial for building a strong mathematical foundation. This article will not only solve the problem but also explore the underlying principles, practical applications, and even some historical context.
Understanding Division and Remainders
Division is one of the four fundamental arithmetic operations, alongside addition, subtraction, and multiplication. It essentially involves splitting a quantity into equal parts. When dividing two whole numbers, we sometimes find that one number doesn't divide evenly into the other. This is where the concept of a remainder comes into play. The remainder is the amount left over after dividing as many times as possible without going into negative numbers.
In the standard notation, we express division as:
Dividend ÷ Divisor = Quotient + Remainder/Divisor
Or, more concisely:
Dividend = (Quotient x Divisor) + Remainder
Let's break down these terms:
- Dividend: The number being divided (in our case, 98).
- Divisor: The number we're dividing by (in our case, 13).
- Quotient: The whole number result of the division (how many times the divisor goes into the dividend).
- Remainder: The amount left over after the division.
Solving 98 Divided by 13
Now let's tackle the problem at hand: 98 divided by 13. We can approach this using several methods:
1. Long Division
Long division is a classic method for performing division, especially when dealing with larger numbers. Here's how it works for 98 ÷ 13:
7
13 | 98
-91
7
- Divide: How many times does 13 go into 98? It goes in 7 times (7 x 13 = 91).
- Multiply: Multiply the quotient (7) by the divisor (13): 7 x 13 = 91.
- Subtract: Subtract the result (91) from the dividend (98): 98 - 91 = 7.
- Remainder: The result of the subtraction (7) is the remainder.
Therefore, 98 divided by 13 is 7 with a remainder of 7.
2. Repeated Subtraction
Another way to visualize division is through repeated subtraction. We repeatedly subtract the divisor from the dividend until we reach a number smaller than the divisor. Let's see:
98 - 13 = 85 85 - 13 = 72 72 - 13 = 59 59 - 13 = 46 46 - 13 = 33 33 - 13 = 20 20 - 13 = 7
We subtracted 13 seven times before reaching a number (7) smaller than 13. This confirms that the quotient is 7 and the remainder is 7.
Practical Applications of Remainders
Remainders aren't just abstract mathematical concepts; they have numerous practical applications in everyday life and various fields:
-
Scheduling and Time Management: Imagine you need to distribute 98 tasks evenly among 13 team members. Each member would get 7 tasks (the quotient), and you would have 7 tasks left over (the remainder) that need to be allocated differently.
-
Counting and Grouping: If you have 98 apples and want to pack them into boxes of 13, you'll fill 7 boxes completely and have 7 apples remaining.
-
Modular Arithmetic (Clock Arithmetic): Remainders are fundamental in modular arithmetic, which is used in cryptography, computer science, and other areas. For example, calculating the time after a certain number of hours, such as what time it is 98 hours after 2 pm, is an application of this.
-
Engineering and Manufacturing: In manufacturing, remainders can represent leftover materials or parts after completing a production run. Efficient management of these remainders is crucial for minimizing waste and optimizing resource utilization.
-
Data Structures and Algorithms: In computer science, the remainder operation (often called the modulo operation, represented by the symbol %) is widely used in algorithms and data structures, such as hashing functions.
Exploring Further: Prime Numbers and Divisibility Rules
The problem of 98 divided by 13 also touches upon the concept of prime numbers and divisibility rules. 13 is a prime number (a number divisible only by 1 and itself). Understanding prime numbers and their properties is essential in various branches of mathematics, including number theory and cryptography.
The lack of a whole number solution for 98 ÷ 13 highlights the fact that not all numbers are divisible by each other. Divisibility rules, which provide shortcuts to determine if a number is divisible by another without performing the full division, can be helpful in situations like this. However, there isn't a straightforward divisibility rule for 13, making long division or repeated subtraction more practical.
Historical Context: The Evolution of Division
Division, as a mathematical operation, has a rich history. Ancient civilizations, including the Babylonians, Egyptians, and Greeks, developed various methods for division. The long division algorithm we use today evolved over centuries, with contributions from mathematicians across different cultures. Understanding the history of division can provide a deeper appreciation for the elegance and efficiency of modern mathematical tools.
Conclusion: Beyond the Numbers
While the answer to 98 divided by 13 is simply 7 with a remainder of 7, this article has demonstrated that this seemingly straightforward problem opens a door to explore a wide range of mathematical concepts, practical applications, and historical context. Mastering division, including understanding remainders, is a critical building block for further mathematical exploration and problem-solving. This extends beyond the classroom, impacting various aspects of our lives, from daily tasks to advanced fields of study. The power of mathematics lies not just in obtaining an answer, but in understanding the underlying principles and appreciating its widespread applications.
Latest Posts
Latest Posts
-
99 Divided By 12 With Remainder
May 15, 2025
-
What Phase Of My Cycle Am I In Calculator
May 15, 2025
-
10 Libras En Kilos Cuanto Es
May 15, 2025
-
1994 Al 2024 Cuantos Anos Son
May 15, 2025
-
What Day Is It 45 Days From Today
May 15, 2025
Related Post
Thank you for visiting our website which covers about 98 Divided By 13 With Remainder . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.