Gcf Of 18 36 And 45
Treneri
May 11, 2025 · 5 min read
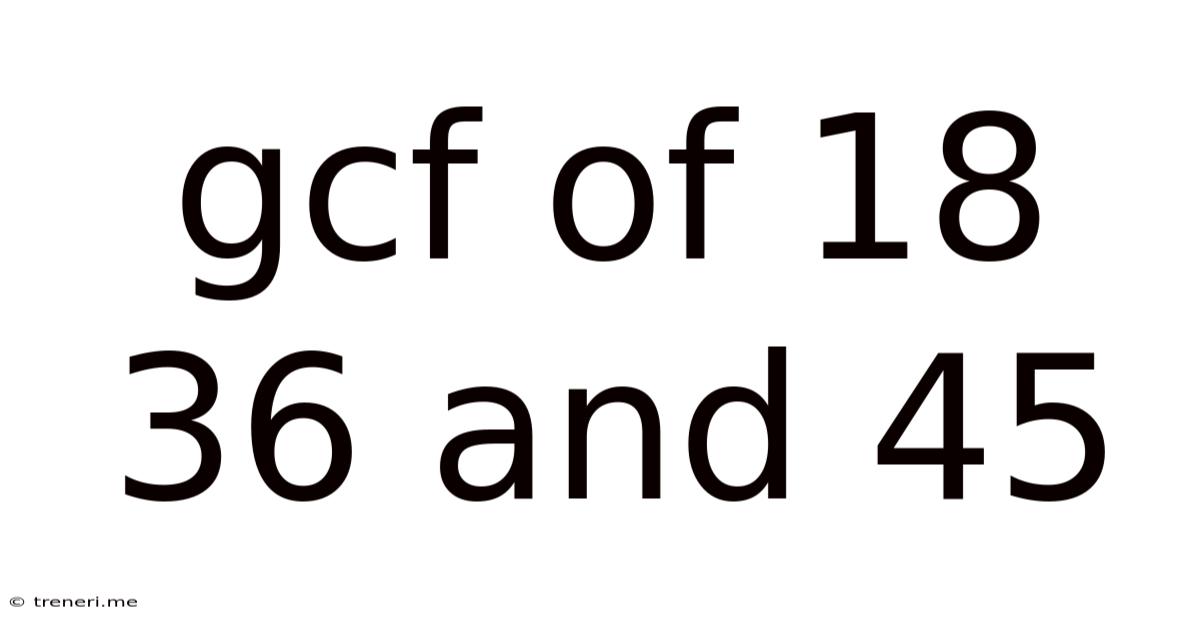
Table of Contents
Finding the Greatest Common Factor (GCF) of 18, 36, and 45: A Comprehensive Guide
Finding the greatest common factor (GCF), also known as the greatest common divisor (GCD), of a set of numbers is a fundamental concept in mathematics with applications in various fields, from simplifying fractions to solving complex algebraic problems. This article delves deep into determining the GCF of 18, 36, and 45, exploring multiple methods and illustrating their practical applications. We'll move beyond simply finding the answer and explore the underlying mathematical principles, ensuring a thorough understanding of this crucial concept.
Understanding the Greatest Common Factor (GCF)
Before we dive into calculating the GCF of 18, 36, and 45, let's solidify our understanding of the term itself. The GCF of a set of numbers is the largest number that divides each number in the set without leaving a remainder. It's the highest common factor shared by all numbers. This is different from the least common multiple (LCM), which is the smallest number that is a multiple of all the numbers in the set.
Why is finding the GCF important?
The GCF has numerous applications across various mathematical areas:
- Simplifying Fractions: Finding the GCF of the numerator and denominator allows for the simplification of fractions to their lowest terms.
- Algebraic Expressions: The GCF is crucial in factoring algebraic expressions, making them easier to solve and analyze.
- Geometry: The GCF helps in solving problems related to area and perimeter calculations involving figures with common dimensions.
- Real-world applications: GCF plays a vital role in scenarios involving equal distribution or grouping items. For example, determining the maximum number of identical gift bags that can be created from a given number of each item.
Methods for Finding the GCF of 18, 36, and 45
Several methods exist for determining the GCF of a set of numbers. We will explore three common approaches:
1. Listing Factors Method
This method involves listing all the factors of each number and then identifying the largest factor common to all.
- Factors of 18: 1, 2, 3, 6, 9, 18
- Factors of 36: 1, 2, 3, 4, 6, 9, 12, 18, 36
- Factors of 45: 1, 3, 5, 9, 15, 45
By comparing the lists, we can see that the common factors are 1, 3, and 9. The largest of these common factors is 9.
Therefore, the GCF of 18, 36, and 45 is 9.
2. Prime Factorization Method
This method involves breaking down each number into its prime factors and then multiplying the common prime factors raised to the lowest power.
- Prime factorization of 18: 2 x 3 x 3 = 2 x 3²
- Prime factorization of 36: 2 x 2 x 3 x 3 = 2² x 3²
- Prime factorization of 45: 3 x 3 x 5 = 3² x 5
The common prime factors are 3. The lowest power of 3 present in all factorizations is 3². Therefore:
GCF(18, 36, 45) = 3² = 9
This method is particularly useful for larger numbers where listing all factors becomes cumbersome.
3. Euclidean Algorithm Method
The Euclidean algorithm is an efficient method, especially for larger numbers, to find the GCF. It's based on the principle that the GCF of two numbers doesn't change if the larger number is replaced by its difference with the smaller number. This process is repeated until the two numbers are equal, and that number is the GCF. To extend this to three or more numbers, we find the GCF of the first two, then find the GCF of that result and the third number, and so on.
Let's apply it to 18, 36, and 45:
-
Find the GCF of 18 and 36:
- 36 - 18 = 18
- 18 - 18 = 0 The GCF(18, 36) = 18
-
Find the GCF of 18 and 45:
- 45 - 18 = 27
- 27 - 18 = 9
- 18 - 9 = 9
- 9 - 9 = 0 The GCF(18, 45) = 9
Therefore, the GCF(18, 36, 45) = 9.
Applications of the GCF of 18, 36, and 45
Understanding the GCF isn't just about abstract mathematical exercises; it has practical implications. Let's explore a few real-world examples:
1. Simplifying Fractions:
Suppose you have the fraction 36/45. Knowing that the GCF(36, 45) = 9, we can simplify the fraction:
36/45 = (36 ÷ 9) / (45 ÷ 9) = 4/5
2. Dividing Objects Equally:
Imagine you have 18 red marbles, 36 blue marbles, and 45 green marbles. You want to divide these marbles into identical bags, with each bag containing the same number of each color marble. The maximum number of bags you can create is determined by the GCF(18, 36, 45) = 9. You can create 9 bags, each containing 2 red, 4 blue, and 5 green marbles.
3. Geometry Problems:
Consider a rectangular garden with dimensions 18 meters by 36 meters. You want to divide the garden into smaller identical square plots. The side length of the largest possible square plot is determined by the GCF(18, 36) = 18 meters. You can create a single row of square plots measuring 18 meters by 18 meters in dimension.
Beyond the Basics: Extending the GCF Concept
The principles of finding the GCF extend to more complex scenarios. Let's briefly explore:
-
GCF of more than three numbers: The methods described above can be easily extended to find the GCF of any number of integers. For the prime factorization and Euclidean algorithm methods, you simply apply the process iteratively.
-
GCF of algebraic expressions: The concept of GCF extends to algebraic expressions. You find the GCF of the coefficients and variables in a similar way to numerical GCF. For example, the GCF of 6x² and 18x is 6x.
-
Applications in cryptography: GCF and related concepts play a role in various cryptographic algorithms, contributing to data security.
Conclusion: Mastering the GCF
Understanding and applying the GCF is a crucial skill in various areas of mathematics and its practical applications. The methods presented – listing factors, prime factorization, and the Euclidean algorithm – provide effective ways to find the GCF, each with its strengths and weaknesses depending on the numbers involved. By mastering these methods, you'll enhance your problem-solving capabilities across many mathematical fields and real-world scenarios. Remember, the key is not just memorizing the steps but understanding the underlying mathematical principles. This ensures you can confidently approach GCF problems regardless of their complexity. So, practice these methods, explore their applications, and deepen your mathematical understanding – the rewards are well worth the effort.
Latest Posts
Latest Posts
-
120 Days From June 20 2024
May 13, 2025
-
Cuanto Es 2000 Ml En Litros
May 13, 2025
-
Convert Litre Per Minute To Cfm
May 13, 2025
-
8 Is 40 Percent Of What Number
May 13, 2025
-
Greatest Common Factor Of 20 And 100
May 13, 2025
Related Post
Thank you for visiting our website which covers about Gcf Of 18 36 And 45 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.