What Fraction Is Equal To 3/6
Treneri
May 09, 2025 · 5 min read
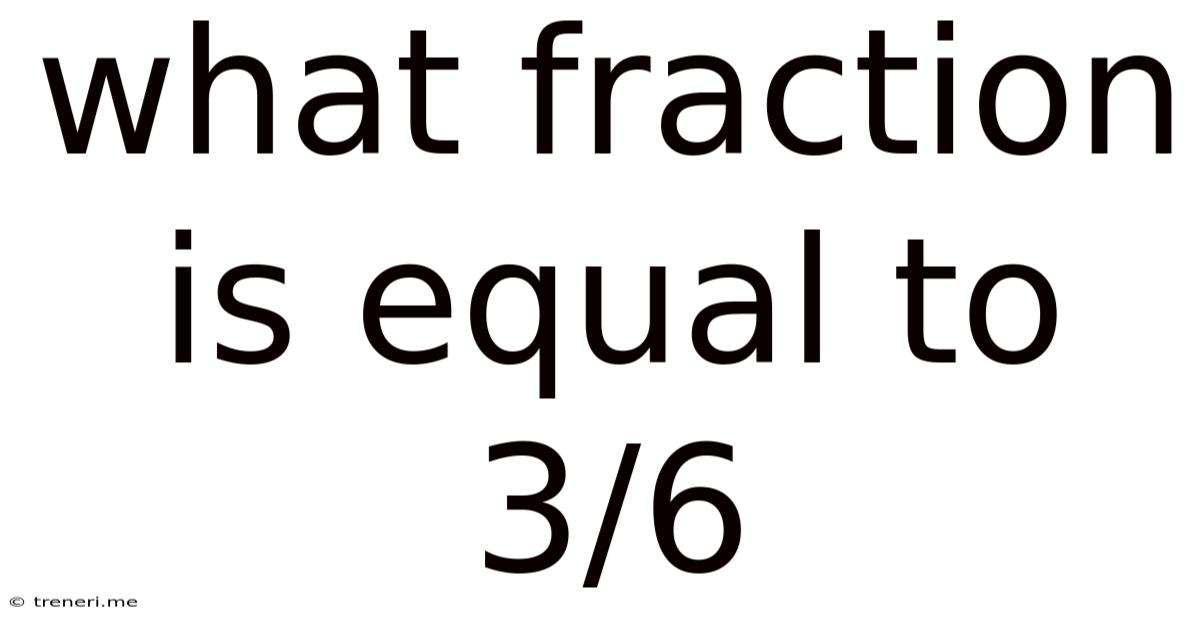
Table of Contents
What Fraction is Equal to 3/6? A Deep Dive into Equivalent Fractions
The question, "What fraction is equal to 3/6?" might seem deceptively simple. It's a fundamental concept in elementary mathematics, yet understanding it thoroughly unlocks a broader understanding of fractions, equivalent fractions, simplification, and even more advanced mathematical concepts. This article will delve deep into this seemingly simple question, exploring the various ways to approach it and expanding on the underlying mathematical principles.
Understanding Fractions: Parts of a Whole
Before we tackle the specific fraction 3/6, let's solidify our understanding of what a fraction represents. A fraction is a way of expressing a part of a whole. It's composed of two key components:
- Numerator: The top number represents the number of parts we are considering.
- Denominator: The bottom number indicates the total number of equal parts the whole is divided into.
For example, in the fraction 3/6, the numerator (3) tells us we're looking at 3 parts, while the denominator (6) tells us the whole is divided into 6 equal parts. Visualizing this with a pie chart or a set of objects makes the concept instantly clearer.
Finding Equivalent Fractions: The Key to Simplification
Equivalent fractions represent the same value, even though they look different. This is because they represent the same proportion of a whole. Think of it like slicing a pizza: you can have one half (1/2) of a pizza, or you could have two quarters (2/4) – both represent the same amount of pizza. Finding equivalent fractions is crucial for simplifying fractions to their simplest form.
To find an equivalent fraction, you simply multiply or divide both the numerator and the denominator by the same non-zero number. This keeps the proportion the same, essentially multiplying or dividing by 1 (e.g., 2/2 = 1 or 5/5 = 1).
Solving the Puzzle: What Fraction is Equal to 3/6?
Now, let's return to our original question: what fraction is equal to 3/6? We can approach this in a few ways:
Method 1: Visualization
Imagine a rectangle divided into six equal parts. Shade three of those parts. Visually, you can see that the shaded portion represents half of the rectangle. This immediately suggests that 3/6 is equivalent to 1/2.
Method 2: Simplification through Division
The most common method to find the equivalent fraction is to simplify the fraction by finding the greatest common divisor (GCD) of the numerator and denominator. The GCD is the largest number that divides both the numerator and the denominator without leaving a remainder.
In the case of 3/6, both 3 and 6 are divisible by 3. Dividing both the numerator and the denominator by 3, we get:
3 ÷ 3 = 1 6 ÷ 3 = 2
Therefore, 3/6 simplifies to 1/2.
Method 3: Finding Equivalent Fractions through Multiplication (Less Efficient for this Example)
While less efficient in this specific case, we can also find equivalent fractions by multiplying both the numerator and the denominator by the same number. For example, multiplying both 1 and 2 (from 1/2) by 3 gives us 3/6. This demonstrates that 1/2 and 3/6 are indeed equivalent.
Why Simplifying Fractions is Important
Simplifying fractions, as we did with 3/6, is crucial for several reasons:
- Clarity: Simplified fractions are easier to understand and compare. 1/2 is inherently simpler and more intuitive than 3/6.
- Efficiency: In calculations involving fractions, simplified fractions make the process significantly less cumbersome.
- Standardization: Having a standard simplified form for a fraction ensures consistent results and avoids confusion.
Expanding on the Concept: Further Exploration of Equivalent Fractions
Understanding the equivalence of 3/6 and 1/2 opens the door to exploring a wide range of related concepts:
Generating Equivalent Fractions: An Infinite Set
For any given fraction, there are infinitely many equivalent fractions. This is because you can always multiply the numerator and denominator by any non-zero number. For example, from 1/2, we can generate:
- 2/4 (multiply by 2/2)
- 3/6 (multiply by 3/3)
- 4/8 (multiply by 4/4)
- and so on...
Comparing Fractions: The Importance of a Common Denominator
When comparing fractions, it is often helpful to find equivalent fractions with a common denominator. This makes it easy to directly compare the numerators. For example, to compare 1/2 and 2/5, we could find equivalent fractions with a denominator of 10:
- 1/2 = 5/10
- 2/5 = 4/10
Now it is clear that 5/10 > 4/10, therefore 1/2 > 2/5.
Fractions and Decimals: Interconversion
Fractions and decimals are two different ways to represent the same value. The fraction 1/2 is equivalent to the decimal 0.5. This interconversion is essential in many mathematical applications. Understanding this relationship helps in solving various problems involving both fractions and decimals.
Fractions and Percentages: Another Interconversion
Similar to decimals, fractions can also be expressed as percentages. 1/2 is equivalent to 50%. This conversion is particularly useful in contexts requiring percentage calculations, like calculating discounts, taxes, or proportions.
Real-World Applications of Equivalent Fractions
Equivalent fractions are not just an abstract mathematical concept; they have practical applications in numerous real-world scenarios:
- Cooking and Baking: Recipes often involve fractions, and understanding equivalent fractions allows for adjustments based on the number of servings needed.
- Construction and Engineering: Precise measurements in these fields heavily rely on fractions and their simplified forms.
- Finance: Calculating interest, proportions, and ratios frequently involves working with fractions.
- Data Analysis: Representing and interpreting data often requires the use of fractions and their equivalent forms.
Conclusion: Mastering the Fundamentals
The seemingly simple question, "What fraction is equal to 3/6?", unveils a wealth of knowledge about fractions, their equivalence, and their practical significance. Mastering the concept of equivalent fractions is fundamental to success in mathematics and its various applications in the real world. Through visualization, simplification techniques, and an understanding of the underlying principles, we can confidently navigate the world of fractions and unlock a deeper appreciation for their importance. Remember, the journey from 3/6 to 1/2 isn't just about simplifying a fraction; it's about grasping a fundamental mathematical concept that empowers you to tackle more complex problems with confidence and clarity.
Latest Posts
Latest Posts
-
Find Area Of Triangle With Apothem
May 10, 2025
-
What Is An Equivalent Fraction For 8 12
May 10, 2025
-
What Is A 3 To 1 Slope
May 10, 2025
-
What Are Two Equivalent Fractions For 3 5
May 10, 2025
-
What Is 3 Percent Of 400
May 10, 2025
Related Post
Thank you for visiting our website which covers about What Fraction Is Equal To 3/6 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.