What Fraction Is Equivalent To 35
Treneri
May 14, 2025 · 5 min read
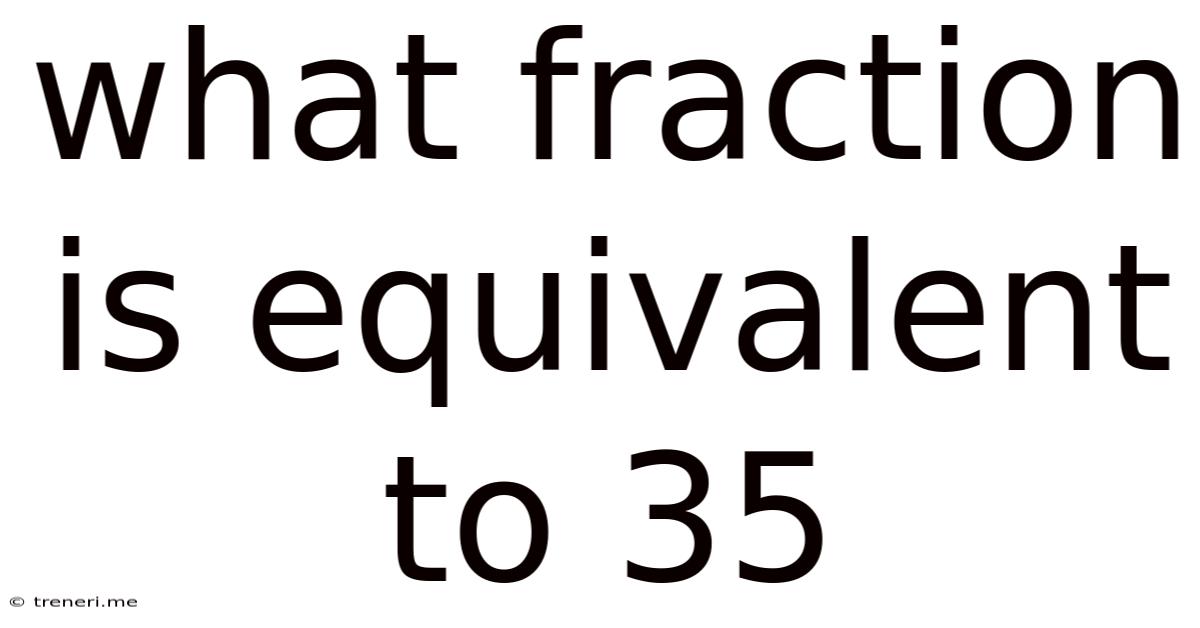
Table of Contents
What Fraction is Equivalent to 35? Understanding Equivalent Fractions and Their Applications
The question "What fraction is equivalent to 35?" might seem deceptively simple at first glance. However, understanding the concept of equivalent fractions and how to find them unlocks a deeper understanding of fundamental mathematical principles and their wide-ranging applications. This article will delve into the intricacies of equivalent fractions, exploring their meaning, how to identify them, and their practical uses in various fields.
Understanding the Concept of Equivalent Fractions
A fraction represents a part of a whole. It's expressed as a ratio of two integers, the numerator (top number) and the denominator (bottom number). Equivalent fractions are fractions that represent the same value, even though they look different. Think of cutting a pizza: 1/2 of a pizza is the same as 2/4 or 3/6, etc. These are all equivalent fractions.
The key to understanding equivalent fractions lies in the concept of proportionality. If we multiply or divide both the numerator and the denominator of a fraction by the same non-zero number, we obtain an equivalent fraction. This is because we're essentially scaling the fraction up or down, maintaining the same relative proportion.
How to Find Equivalent Fractions
Let's examine several methods for finding fractions equivalent to a given fraction. We'll use the example of 3/5 to illustrate these methods. Remember that 35 in the original question needs clarification. It seems to be a typo, likely representing 3/5 or a similar fraction. For now, we will proceed with 3/5 as the given fraction.
1. Multiplying the Numerator and Denominator by the Same Number:
This is the most straightforward approach. Choose any non-zero integer (let's use 2, 3, and 4 as examples) and multiply both the numerator and the denominator of 3/5 by that integer:
- Multiplying by 2: (3 x 2) / (5 x 2) = 6/10
- Multiplying by 3: (3 x 3) / (5 x 3) = 9/15
- Multiplying by 4: (3 x 4) / (5 x 4) = 12/20
All of these fractions – 6/10, 9/15, and 12/20 – are equivalent to 3/5. They represent the same proportion or part of a whole.
2. Dividing the Numerator and Denominator by the Same Number (Simplification):
If we start with a larger fraction, we can find an equivalent fraction by simplifying it. This involves finding the greatest common divisor (GCD) of the numerator and denominator and dividing both by the GCD. This process finds the simplest form of the fraction, also known as its lowest terms.
For example, let's consider the fraction 12/20. The GCD of 12 and 20 is 4. Dividing both the numerator and denominator by 4:
(12 ÷ 4) / (20 ÷ 4) = 3/5
This confirms that 12/20 is indeed equivalent to 3/5. Simplification ensures that the fraction is expressed in its most concise and manageable form.
3. Using Visual Aids:
Visual representations can significantly aid in understanding equivalent fractions. Imagine a rectangular shape divided into five equal parts. Shade three of them to represent 3/5. Now, imagine dividing each of the five parts into two smaller, equal parts. You now have ten parts, and six are shaded (6/10), maintaining the same proportion as 3/5.
Applications of Equivalent Fractions
The concept of equivalent fractions extends far beyond basic arithmetic. They are fundamental in various mathematical and practical applications:
-
Simplifying Calculations: Working with simplified fractions makes calculations easier and less prone to errors. Imagine adding 12/20 and 6/10. Simplifying 12/20 to 3/5 makes the addition far simpler (3/5 + 6/10 = 12/10 = 6/5).
-
Comparing Fractions: Equivalent fractions facilitate easy comparison of fractions with different denominators. To compare 3/5 and 2/3, we can find equivalent fractions with a common denominator (e.g., 15): 3/5 = 9/15 and 2/3 = 10/15. This immediately shows that 2/3 is greater than 3/5.
-
Ratio and Proportion Problems: Equivalent fractions are crucial in solving problems involving ratios and proportions. These problems arise frequently in fields like cooking (scaling recipes), construction (measuring materials), and many other real-world scenarios.
-
Algebra and Equation Solving: Understanding equivalent fractions is vital in solving algebraic equations involving fractions. Finding common denominators or simplifying expressions often relies on the manipulation of equivalent fractions.
-
Geometry and Measurement: Equivalent fractions play a role in geometric calculations, particularly in finding the area and volume of shapes. Expressing different measurements using equivalent fractions can simplify calculations and ensure consistent units.
-
Data Analysis and Probability: When analyzing data or calculating probabilities, working with equivalent fractions simplifies the interpretation and presentation of results. Reducing fractions to their simplest form ensures clarity and efficiency.
Advanced Concepts Related to Equivalent Fractions
The concept of equivalent fractions leads to more advanced mathematical ideas:
-
Rational Numbers: The set of rational numbers encompasses all numbers that can be expressed as a fraction of two integers (where the denominator is not zero). Equivalent fractions highlight the fact that a single rational number can have multiple fractional representations.
-
Decimal Representation: Every fraction can be represented as a decimal number (by performing division). Equivalent fractions share the same decimal representation (or have decimal representations that differ only in the number of trailing zeros).
-
Continued Fractions: A continued fraction is an expression obtained by repeatedly applying the Euclidean algorithm to a rational number. Understanding equivalent fractions helps in analyzing and manipulating these continued fractions.
Addressing Potential Misunderstandings
A common error is to add or subtract the numerators and denominators separately when dealing with equivalent fractions. Remember, this is incorrect. To find an equivalent fraction, you must multiply or divide both the numerator and the denominator by the same non-zero number.
Conclusion
The seemingly simple question of finding fractions equivalent to 3/5 (assuming the original 35 was a typo) reveals a deep and important mathematical concept with far-reaching applications. Understanding equivalent fractions is not just about manipulating numbers; it's about grasping the underlying principles of proportionality, ratio, and representation. This understanding forms a crucial foundation for more advanced mathematical concepts and problem-solving in various fields, from cooking to engineering. Mastering this concept empowers individuals to approach a wide range of problems with greater confidence and efficiency. So, the next time you encounter a fraction, remember the power and versatility of equivalent fractions and their significant role in making mathematical calculations easier and more intuitive.
Latest Posts
Latest Posts
-
How Many Calories Do I Burn Sitting Calculator
May 14, 2025
-
How Many Weeks Is 13 Years
May 14, 2025
-
1 8 Per Foot Slope In Percent
May 14, 2025
-
What Is The Greatest Common Factor Of 42 And 70
May 14, 2025
-
What Percent Of 21 Is 7
May 14, 2025
Related Post
Thank you for visiting our website which covers about What Fraction Is Equivalent To 35 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.