What Is 1/4 + 3/8 Reduced To The Lowest Terms
Treneri
May 11, 2025 · 5 min read
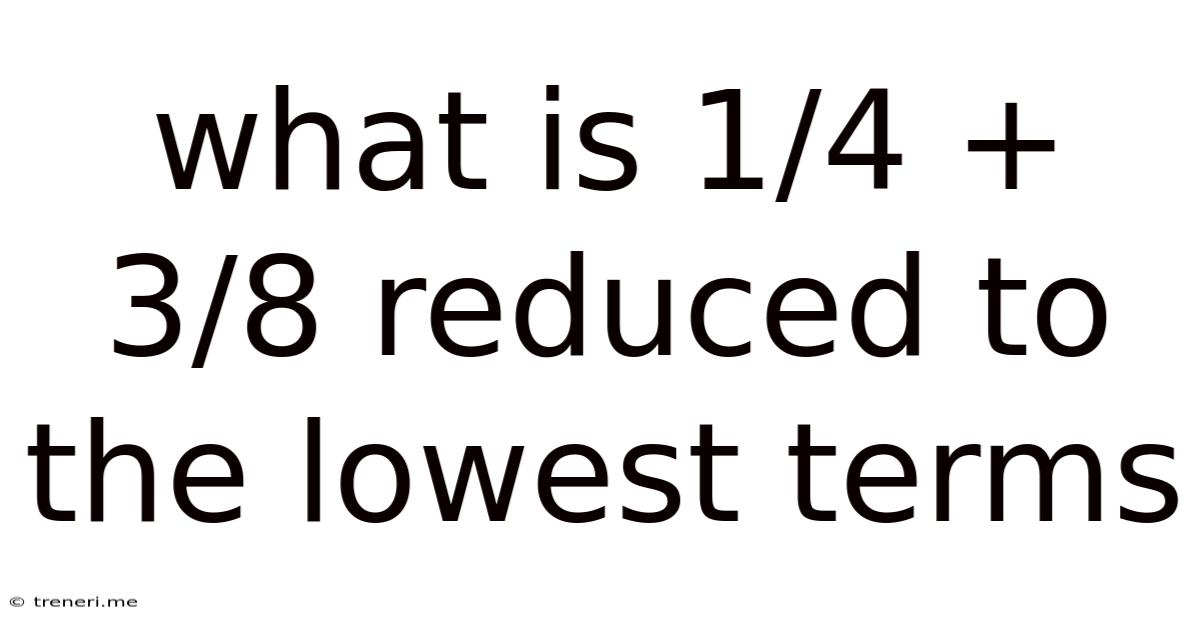
Table of Contents
What is 1/4 + 3/8 Reduced to the Lowest Terms? A Comprehensive Guide to Fraction Addition and Simplification
Adding fractions might seem like a simple arithmetic task, but understanding the underlying principles and mastering the techniques involved is crucial for various mathematical applications. This comprehensive guide will delve into the process of adding 1/4 and 3/8, simplifying the result to its lowest terms, and exploring the broader concepts of fraction addition and simplification.
Understanding Fractions
Before we tackle the problem, let's refresh our understanding of fractions. A fraction represents a part of a whole. It's composed of two main parts:
- Numerator: The top number, indicating the number of parts we have.
- Denominator: The bottom number, indicating the total number of equal parts the whole is divided into.
For example, in the fraction 1/4, the numerator is 1 (we have one part), and the denominator is 4 (the whole is divided into four equal parts).
Finding a Common Denominator
Adding fractions requires a crucial step: finding a common denominator. This is a number that is a multiple of both denominators. Why is this necessary? Imagine trying to add apples and oranges – you need a common unit to combine them. Similarly, we need a common denominator to add fractions with different denominators.
In our problem, 1/4 + 3/8, the denominators are 4 and 8. Let's find the least common multiple (LCM) of 4 and 8. The multiples of 4 are 4, 8, 12, 16… The multiples of 8 are 8, 16, 24… The least common multiple is 8.
Converting Fractions to Equivalent Fractions
Now that we have a common denominator (8), we need to convert each fraction to an equivalent fraction with a denominator of 8. This means we need to find fractions that have the same value as the original fractions but with the denominator 8.
-
Converting 1/4: To change the denominator from 4 to 8, we multiply both the numerator and denominator by 2: (1 x 2) / (4 x 2) = 2/8. This doesn't change the value of the fraction; it simply represents the same proportion in a different form.
-
3/8: This fraction already has a denominator of 8, so we don't need to change it.
Adding the Fractions
Now that both fractions have the same denominator, we can add them:
2/8 + 3/8 = (2 + 3) / 8 = 5/8
Reducing to Lowest Terms (Simplifying)
The result, 5/8, is a fraction. However, it's important to always simplify fractions to their lowest terms. This means finding the greatest common divisor (GCD) of the numerator and denominator and dividing both by it.
The factors of 5 are 1 and 5. The factors of 8 are 1, 2, 4, and 8.
The greatest common divisor of 5 and 8 is 1. Since the GCD is 1, the fraction 5/8 is already in its lowest terms; we cannot simplify it further.
Therefore, 1/4 + 3/8 reduced to the lowest terms is 5/8.
Deeper Dive into Fraction Simplification
Simplifying fractions to their lowest terms is essential for several reasons:
-
Clarity: Simplified fractions are easier to understand and interpret. 5/8 is clearer than, say, 10/16, even though they represent the same value.
-
Comparison: Comparing fractions is simpler when they're in their lowest terms. It's easier to compare 5/8 and 3/4 than 10/16 and 12/16.
-
Calculations: In more complex calculations involving fractions, working with simplified fractions makes the process smoother and less prone to errors.
-
Efficiency: Simplified fractions represent the most efficient and concise way to express a fractional value.
Practical Applications of Fraction Addition and Simplification
Understanding fraction addition and simplification isn't just an academic exercise; it has numerous practical applications in everyday life and various fields:
-
Cooking and Baking: Recipes often involve fractional measurements. Adding fractions is crucial to calculate the total amount of ingredients.
-
Construction and Engineering: Accurate measurements are critical, and fractions are often used to express precise dimensions.
-
Finance: Understanding fractions is essential for dealing with percentages, interest rates, and proportions in financial calculations.
-
Data Analysis: Fractions and ratios are frequently used to represent data proportions and relationships.
-
Science: Fractions and ratios are fundamental in scientific calculations and measurements.
Advanced Concepts Related to Fraction Addition
This section expands on the fundamental concepts, introducing related topics to provide a more complete understanding.
Adding More Than Two Fractions:
The process extends seamlessly to adding more than two fractions. First, find the least common denominator for all the fractions. Then, convert each fraction to an equivalent fraction with that common denominator. Finally, add the numerators and keep the common denominator. Remember to simplify the resulting fraction to its lowest terms.
Mixed Numbers:
A mixed number combines a whole number and a fraction (e.g., 1 1/2). To add mixed numbers, you can either convert them to improper fractions (where the numerator is greater than the denominator) first, then follow the standard fraction addition procedure, or add the whole numbers and fractions separately, then combine the results.
Subtracting Fractions:
Subtracting fractions follows a similar process to adding fractions. Find a common denominator, convert the fractions, subtract the numerators, and simplify the result. Be mindful of borrowing if the numerator of the fraction being subtracted is larger than the numerator of the fraction it's being subtracted from.
Multiplying and Dividing Fractions:
While this article focuses on addition, multiplying and dividing fractions have distinct rules. Multiplying involves multiplying the numerators and denominators separately. Dividing involves inverting the second fraction (reciprocal) and then multiplying.
Conclusion
Adding 1/4 and 3/8, and simplifying the result to 5/8, is a fundamental concept in arithmetic. Mastering this skill involves understanding fractions, finding common denominators, converting to equivalent fractions, adding the numerators, and simplifying to the lowest terms. This skill has far-reaching implications in various aspects of life, from everyday tasks to complex calculations in various professional fields. A solid grasp of these principles is essential for anyone seeking to develop a strong foundation in mathematics. Further exploration into the more advanced concepts discussed will solidify your understanding and proficiency in working with fractions.
Latest Posts
Latest Posts
-
Compute The Volume Of The Right Square Pyramid Shown
May 12, 2025
-
What Fraction Is Equal To 2 5
May 12, 2025
-
638 Rounded To The Nearest Hundred
May 12, 2025
-
What Is 90 Days From July 15 2024
May 12, 2025
-
What Are Equivalent Fractions For 5 6
May 12, 2025
Related Post
Thank you for visiting our website which covers about What Is 1/4 + 3/8 Reduced To The Lowest Terms . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.