What Is 9 Percent Of 20
Treneri
May 11, 2025 · 5 min read
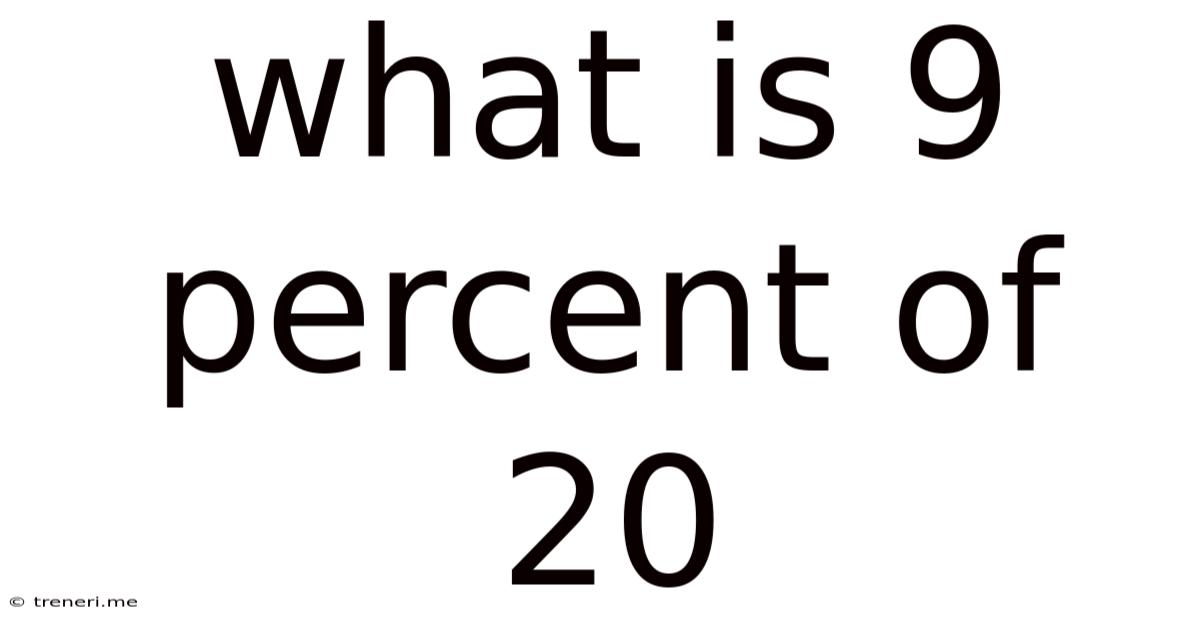
Table of Contents
What is 9 Percent of 20? A Deep Dive into Percentage Calculations
Finding 9 percent of 20 might seem like a simple calculation, but it opens the door to understanding a fundamental concept in mathematics and its widespread applications in everyday life. This article will not only answer the question directly but will also explore the underlying principles, various methods of calculation, and real-world examples to solidify your understanding of percentages.
Understanding Percentages
A percentage is a fraction or ratio expressed as a number out of 100. The term "percent" literally means "per hundred." When we say "9 percent," we mean 9 out of every 100. This can be represented as a fraction (9/100) or a decimal (0.09). Understanding this fundamental concept is key to tackling any percentage problem.
Key Terms:
- Percentage: The portion of a whole expressed as a number out of 100.
- Base: The whole amount or total value. In our problem, the base is 20.
- Rate: The percentage expressed as a decimal or fraction. In our case, the rate is 9% or 0.09.
- Part: The result of finding the percentage of the base. This is what we are trying to calculate.
Calculating 9 Percent of 20: Three Methods
There are several ways to calculate 9 percent of 20. Let's explore three common methods:
Method 1: Using the Decimal Equivalent
This is perhaps the most straightforward method. We convert the percentage to its decimal equivalent and then multiply it by the base:
- Convert the percentage to a decimal: 9% = 9/100 = 0.09
- Multiply the decimal by the base: 0.09 * 20 = 1.8
Therefore, 9 percent of 20 is $\boxed{1.8}$
Method 2: Using the Fraction Equivalent
We can also use the fraction equivalent of 9 percent:
- Convert the percentage to a fraction: 9% = 9/100
- Multiply the fraction by the base: (9/100) * 20 = 180/100 = 1.8
This method yields the same result: 9 percent of 20 is $\boxed{1.8}$
Method 3: Proportion Method
This method is particularly useful for understanding the underlying relationship between the percentage, the base, and the part. We set up a proportion:
- Let x represent the unknown part (9% of 20).
- Set up the proportion: 9/100 = x/20
- Cross-multiply: 9 * 20 = 100 * x
- Solve for x: 180 = 100x => x = 180/100 = 1.8
Once again, we find that 9 percent of 20 is $\boxed{1.8}$
Real-World Applications of Percentage Calculations
Understanding percentage calculations is crucial in various real-world scenarios:
1. Sales Tax and Discounts:
Imagine you're buying an item priced at $20, and the sales tax is 9%. To calculate the tax amount, you'd find 9% of $20, which, as we've established, is $1.80. Similarly, if an item is discounted by 9%, you would subtract 9% of the original price from the original price.
2. Tips and Gratuities:
In restaurants, it's customary to leave a tip. If you want to leave a 9% tip on a $20 meal, you would calculate 9% of $20, resulting in a $1.80 tip.
3. Interest Calculations:
Interest on loans and savings accounts is often calculated as a percentage of the principal amount. Understanding percentage calculations is vital for comprehending interest accrual and loan repayments. For example, if you have a savings account with a 9% annual interest rate and $20 in the account, you would earn $1.80 in interest in a year.
4. Grade Calculations:
In many educational systems, grades are often expressed as percentages. Understanding percentages helps students interpret their academic performance and track their progress.
5. Statistical Analysis:
Percentages play a critical role in statistical analysis, allowing us to represent data as proportions and make comparisons. For instance, if 9% of a survey's respondents answered a question in a specific way, this represents 9 out of every 100 respondents.
6. Financial Planning:
Percentage calculations are essential for budgeting, investment strategies, and retirement planning. Understanding how percentages affect your income, expenses, and investment returns is vital for making informed financial decisions.
Beyond the Basics: More Complex Percentage Problems
While calculating 9% of 20 is relatively simple, the principles involved can be extended to more complex scenarios:
- Finding the percentage increase or decrease: If a value increases or decreases by a certain percentage, you can use percentage calculations to determine the new value.
- Calculating the original value from a percentage: If you know a percentage of a value and the resulting amount, you can work backward to find the original value.
- Compound interest: Compound interest involves calculating interest on both the principal amount and accumulated interest. This requires repeated percentage calculations.
Mastering Percentages: Tips and Resources
Mastering percentage calculations requires practice. Here are some tips:
- Practice regularly: Work through various percentage problems to build your proficiency.
- Use multiple methods: Try different approaches to solve problems, reinforcing your understanding.
- Utilize online calculators and resources: These tools can be helpful for checking your work and exploring more complex problems. However, it's crucial to understand the underlying principles before relying solely on calculators.
- Break down complex problems: Decompose intricate percentage problems into smaller, manageable steps.
Conclusion
Calculating 9 percent of 20 – which is 1.8 – is a seemingly simple task but reveals the power and versatility of percentage calculations. This fundamental mathematical concept has vast real-world applications, impacting various aspects of our lives from everyday purchases to complex financial decisions. By understanding the different methods of calculation and practicing regularly, you can confidently tackle various percentage-related problems and enhance your numerical literacy. Remember, the key is to grasp the underlying principles – the relationship between the percentage, the base, and the part – and apply them consistently.
Latest Posts
Latest Posts
-
Whats The Square Root Of 1 9
May 12, 2025
-
What Is 1 2 As An Improper Fraction
May 12, 2025
-
2 7 Kg To Lbs And Oz
May 12, 2025
-
How Many Days Is 39 Years
May 12, 2025
-
How Many Square Feet In One Bundle Of Roofing Shingles
May 12, 2025
Related Post
Thank you for visiting our website which covers about What Is 9 Percent Of 20 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.