What Is The Square Root Of 47
Treneri
Apr 05, 2025 · 5 min read
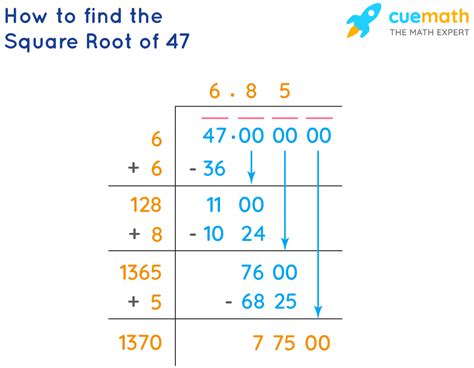
Table of Contents
What is the Square Root of 47? A Deep Dive into Irrational Numbers
The seemingly simple question, "What is the square root of 47?" opens a fascinating door into the world of mathematics, specifically the realm of irrational numbers. While a quick calculator search will give you a decimal approximation, understanding the nature of the square root of 47 requires a deeper exploration. This article will not only provide the answer but delve into the concepts surrounding irrational numbers, methods of approximation, and the historical context of square roots.
Understanding Square Roots
Before we tackle the square root of 47, let's establish a foundational understanding of square roots. The square root of a number (x) is a value (y) that, when multiplied by itself, equals x. Mathematically, this is represented as: y² = x, and y = √x. For example, the square root of 9 (√9) is 3 because 3 * 3 = 9.
Why 47 is Special: The Realm of Irrational Numbers
Unlike the square root of 9, the square root of 47 is not a whole number. It's what we call an irrational number. Irrational numbers cannot be expressed as a simple fraction (a ratio of two integers). Their decimal representation goes on forever without repeating. This is a fundamental difference from rational numbers, like 1/2 or 0.75, which can be expressed as a fraction and have either a terminating or repeating decimal expansion.
The square root of 47 falls into this category of infinite, non-repeating decimals. This means we can only approximate its value.
Approximating √47: Methods and Techniques
Several methods can approximate the square root of 47. Let's explore a few:
1. Using a Calculator: The Quickest Method
The easiest way to get an approximation is by using a calculator. Most calculators will provide a value around 6.8556546. This is a decimal approximation accurate to several decimal places. However, it's important to remember that this is just an approximation, not the exact value.
2. The Babylonian Method (or Heron's Method): An Iterative Approach
This ancient method provides a way to iteratively refine an approximation. It involves starting with an initial guess and repeatedly refining it using a formula:
x_(n+1) = 0.5 * (x_n + (N / x_n))
Where:
- x_n is the current approximation
- x_(n+1) is the improved approximation
- N is the number you're finding the square root of (in this case, 47)
Let's illustrate this with a few iterations:
- Iteration 1: Let's start with an initial guess of x_1 = 7 (because 7 * 7 = 49, close to 47). x_2 = 0.5 * (7 + (47 / 7)) ≈ 6.857
- Iteration 2: Using x_2 as our new guess: x_3 = 0.5 * (6.857 + (47 / 6.857)) ≈ 6.85565
- Iteration 3: Continuing the process will yield even greater accuracy.
As you can see, this method converges towards the actual value quite quickly.
3. Linear Approximation: A Simpler, Less Accurate Method
A simpler, but less accurate, method involves using a linear approximation. We can use the known square roots of numbers close to 47, such as √49 = 7 and √36 = 6. Since 47 lies between 36 and 49, its square root will be between 6 and 7. A rough linear interpolation might give us a value around 6.8. This is a very basic approximation, and its accuracy is limited.
The Significance of Irrational Numbers
The existence of irrational numbers like √47 was a groundbreaking discovery in ancient mathematics. It challenged the prevailing notion that all numbers could be expressed as ratios. The discovery of irrational numbers had significant implications for the development of geometry and calculus. It highlighted the limitations of representing all numbers with simple fractions and paved the way for a deeper understanding of the real number system.
√47 in Different Contexts: Applications and Significance
While √47 might not seem to have immediate practical applications in everyday life, it plays a role in various mathematical and scientific contexts:
-
Geometry: Calculations involving triangles, circles, and other shapes frequently use square roots. If a right-angled triangle has sides of a certain length, the length of the hypotenuse (the longest side) may involve a square root like √47.
-
Physics: Many physical phenomena, like calculating velocity, acceleration, or wave functions, involve square roots.
-
Engineering: Square roots are essential in various engineering applications, such as structural analysis, electrical circuit calculations, and more.
-
Computer Graphics: Calculations involving 3D graphics and modeling frequently use square roots.
-
Statistics: Calculations of standard deviation frequently involve square roots.
Historical Context: The Search for Square Roots
The study of square roots dates back to ancient civilizations. Babylonian mathematicians developed sophisticated methods for approximating square roots, as evidenced by clay tablets containing their calculations. The Greeks, notably Pythagoras, also made significant contributions. The discovery of irrational numbers challenged their understanding of numbers and prompted further mathematical advancements.
Beyond the Calculation: Understanding the Concept
The importance of understanding the square root of 47 extends beyond simply obtaining a numerical answer. It's about grasping the concept of irrational numbers and their significance within the broader field of mathematics. It reinforces the understanding that numbers exist beyond simple fractions and highlights the complexity and beauty of the mathematical world. It's a stepping stone to understanding more advanced mathematical concepts.
Conclusion: More Than Just a Number
The square root of 47, while seemingly a simple calculation, reveals a deeper understanding of mathematics. It's a prime example of an irrational number, showcasing the infinite, non-repeating nature of its decimal representation. Understanding how to approximate its value through various methods underscores the power of mathematical tools and techniques. Its significance extends beyond a simple numerical answer, emphasizing its role in geometry, physics, engineering, and other fields. By exploring this seemingly simple concept, we gain a richer appreciation for the elegance and complexity of the mathematical world. The pursuit of understanding √47 opens up a broader perspective on the fascinating world of irrational numbers and their importance in various scientific and mathematical disciplines.
Latest Posts
Latest Posts
-
What Day Is 120 Days From Now
Apr 06, 2025
-
Dry To Cooked Pasta Weight Calculator
Apr 06, 2025
-
10 To The Negative 6th Power
Apr 06, 2025
-
Cuanto Es Una Onza En Peso
Apr 06, 2025
-
How Many Mcg Is 5000 Iu
Apr 06, 2025
Related Post
Thank you for visiting our website which covers about What Is The Square Root Of 47 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
