What Percent Is 16 Of 250
Treneri
Apr 24, 2025 · 4 min read
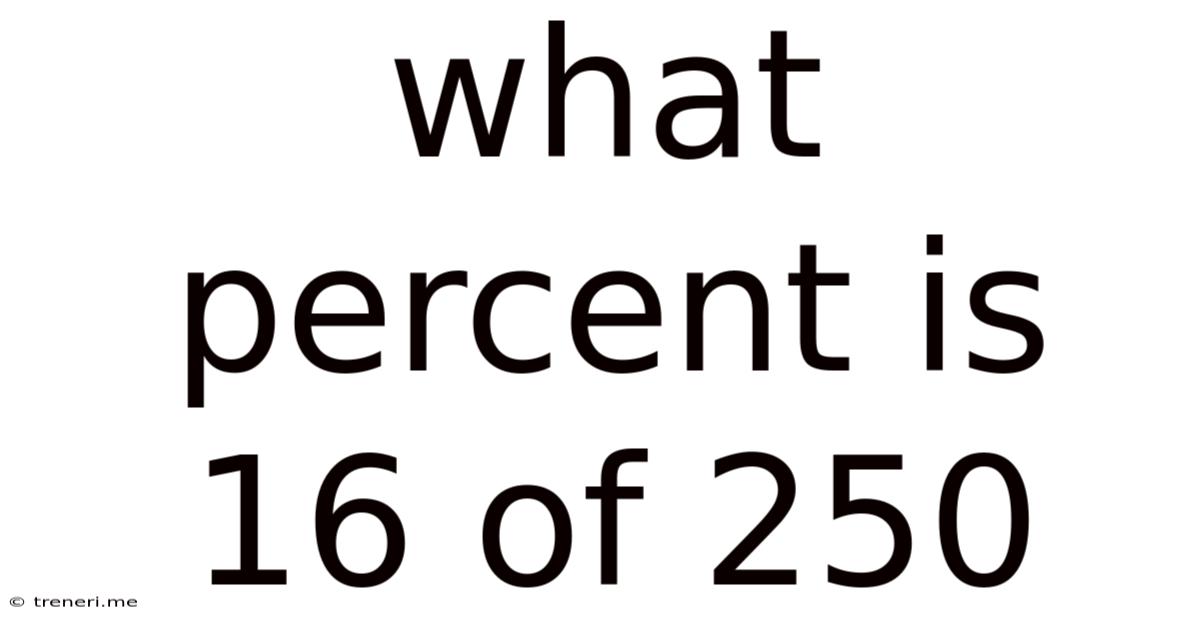
Table of Contents
What Percent is 16 of 250? A Deep Dive into Percentage Calculations
Calculating percentages is a fundamental skill in numerous aspects of life, from understanding financial reports and sale discounts to analyzing statistical data and assessing academic performance. This comprehensive guide will not only answer the question "What percent is 16 of 250?" but also equip you with the knowledge and tools to tackle similar percentage problems with confidence. We'll explore various methods, delve into the underlying concepts, and provide practical applications to solidify your understanding.
Understanding Percentages: The Basics
Before we jump into the specific calculation, let's refresh our understanding of percentages. A percentage is simply a fraction expressed as a number out of 100. The symbol "%" represents "per hundred" or "out of 100." For example, 50% means 50 out of 100, which is equivalent to the fraction 50/100 or the decimal 0.5.
Method 1: The Proportion Method
This method uses the concept of proportions to solve percentage problems. We can set up a proportion to find the percentage:
- Part/Whole = Percentage/100
In our case, the part is 16, the whole is 250, and we want to find the percentage. Let's represent the unknown percentage as 'x':
- 16/250 = x/100
To solve for x, we cross-multiply:
- 16 * 100 = 250 * x
- 1600 = 250x
Now, divide both sides by 250:
- x = 1600 / 250
- x = 6.4
Therefore, 16 is 6.4% of 250.
Method 2: The Decimal Method
This method involves converting the fraction to a decimal and then multiplying by 100 to obtain the percentage.
- Form the fraction: Represent the problem as a fraction: 16/250
- Convert to decimal: Divide 16 by 250: 16 ÷ 250 = 0.064
- Convert to percentage: Multiply the decimal by 100: 0.064 * 100 = 6.4%
This confirms that 16 is 6.4% of 250.
Method 3: Using a Calculator
Most calculators have a percentage function that simplifies this calculation. Simply enter 16 ÷ 250 and then multiply the result by 100. The calculator will directly display the answer as 6.4%. This method is particularly useful for larger or more complex numbers.
Practical Applications and Real-World Examples
Understanding percentage calculations is crucial in many real-world scenarios. Here are a few examples:
-
Sales and Discounts: If a store offers a 20% discount on an item priced at $250, the discount amount is 20% of $250, which is (20/100) * $250 = $50. The final price would be $250 - $50 = $200.
-
Financial Analysis: Percentage calculations are essential when analyzing financial statements. For instance, calculating profit margins, return on investment (ROI), and debt-to-equity ratios all involve percentage calculations.
-
Academic Performance: Grade percentages are a common way to represent academic performance. If a student scores 16 out of 250 points on a test, their percentage score is 6.4%, as calculated above.
-
Statistical Analysis: Percentages are used extensively in statistical analysis to represent proportions, frequencies, and probabilities. For instance, expressing survey results, population demographics, or experimental outcomes often uses percentages.
-
Scientific Research: Percentage change is frequently employed in scientific research to analyze changes over time or to compare different groups. For example, the percentage increase or decrease in a certain population.
Expanding Your Understanding: More Complex Percentage Problems
While the example of 16 out of 250 is relatively straightforward, the principles discussed can be applied to more complex problems. Let's consider some variations:
-
Finding the whole: If you know the percentage (e.g., 6.4%) and the part (e.g., 16), you can calculate the whole. The formula would be: Whole = Part / (Percentage/100).
-
Finding the part: If you know the percentage (e.g., 6.4%) and the whole (e.g., 250), you can calculate the part. The formula would be: Part = (Percentage/100) * Whole.
-
Percentage change: This involves calculating the percentage increase or decrease between two values. The formula is: [(New Value - Old Value) / Old Value] * 100.
Mastering Percentages: Tips and Tricks
-
Practice regularly: The more you practice, the more comfortable you'll become with percentage calculations. Try solving various problems with different numbers.
-
Use different methods: Familiarize yourself with all the methods discussed (proportion, decimal, calculator) to find the approach that works best for you.
-
Break down complex problems: If a problem seems overwhelming, break it down into smaller, more manageable steps.
-
Check your work: Always double-check your calculations to ensure accuracy.
Conclusion: Beyond the Numbers
This in-depth exploration of percentage calculations has not only answered the initial question, "What percent is 16 of 250?" (6.4%), but has also provided a comprehensive understanding of this fundamental mathematical concept. Mastering percentages empowers you to confidently tackle various challenges across numerous fields, from personal finance to scientific research. By applying the methods and techniques described, you can enhance your analytical skills and improve your problem-solving capabilities. Remember that consistent practice and a deep understanding of the underlying principles are key to becoming proficient in percentage calculations. So, keep practicing, and you’ll soon find yourself effortlessly navigating the world of percentages!
Latest Posts
Latest Posts
-
How Many Sides Has A Octagon
May 09, 2025
-
What Is The Gcf Of 15 And 18
May 09, 2025
-
What Is 120 Days Before Today
May 09, 2025
-
Whats 5 To The Power Of 2
May 09, 2025
-
How Many Oz Is 800 Grams
May 09, 2025
Related Post
Thank you for visiting our website which covers about What Percent Is 16 Of 250 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.