What Percentage Is 4 Out Of 24
Treneri
May 09, 2025 · 5 min read
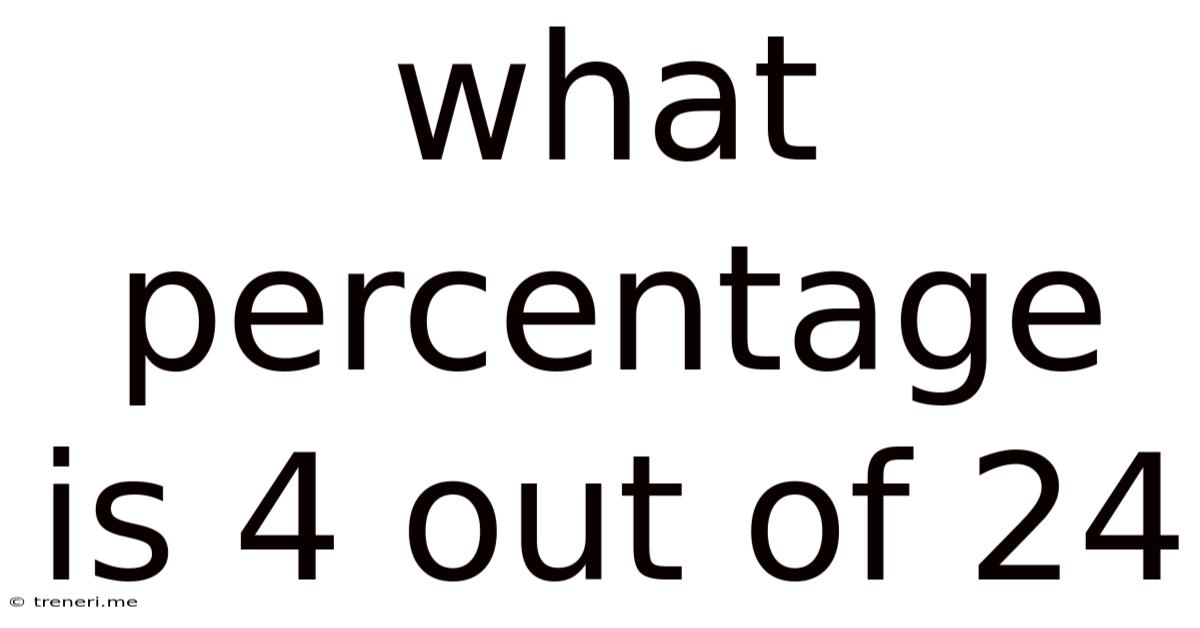
Table of Contents
What Percentage is 4 out of 24? A Comprehensive Guide to Percentage Calculations
Understanding percentages is a fundamental skill in numerous aspects of life, from calculating discounts and tax rates to analyzing data and understanding statistics. This comprehensive guide will delve into the question, "What percentage is 4 out of 24?" and will equip you with the knowledge and techniques to confidently tackle similar percentage problems. We'll cover various methods, explore practical applications, and offer tips and tricks to master percentage calculations.
Understanding Percentages
A percentage is a fraction or ratio expressed as a number out of 100. The symbol "%" signifies "per cent," meaning "out of one hundred." For example, 50% means 50 out of 100, which is equivalent to the fraction 50/100 or the decimal 0.5.
Calculating "What Percentage is 4 out of 24?"
There are several ways to calculate what percentage 4 represents out of 24. Let's explore the most common methods:
Method 1: Using the Fraction Method
-
Express the numbers as a fraction: The problem "What percentage is 4 out of 24?" can be expressed as the fraction 4/24.
-
Simplify the fraction: Reduce the fraction to its simplest form by finding the greatest common divisor (GCD) of the numerator (4) and the denominator (24). The GCD of 4 and 24 is 4. Divide both the numerator and the denominator by 4:
4/24 = 1/6
-
Convert the fraction to a decimal: Divide the numerator (1) by the denominator (6):
1 ÷ 6 ≈ 0.1667
-
Convert the decimal to a percentage: Multiply the decimal by 100 and add the percentage symbol (%):
0.1667 x 100 ≈ 16.67%
Therefore, 4 out of 24 is approximately 16.67%.
Method 2: Using the Proportion Method
This method involves setting up a proportion:
- Part/Whole = Percentage/100
-
Identify the parts: The "part" is 4, and the "whole" is 24.
-
Set up the proportion:
4/24 = x/100
-
Solve for x: Cross-multiply and solve for x:
24x = 400 x = 400/24 x ≈ 16.67
Therefore, x ≈ 16.67%.
Method 3: Using a Calculator
Most calculators have a percentage function. Simply divide 4 by 24 and then multiply the result by 100:
(4 ÷ 24) x 100 ≈ 16.67%
Practical Applications of Percentage Calculations
Understanding percentage calculations is crucial in various real-world scenarios:
1. Finance and Budgeting:
- Calculating discounts: If a store offers a 20% discount on an item, you can use percentage calculations to determine the final price.
- Understanding interest rates: Interest rates on loans and savings accounts are expressed as percentages.
- Analyzing financial statements: Percentage changes in revenue, expenses, and profits are essential for financial analysis.
2. Data Analysis and Statistics:
- Calculating percentages of a population: Determining the percentage of a population that possesses certain characteristics is vital for market research, public health studies, and social science analysis.
- Interpreting survey results: Survey data is frequently presented as percentages to show the proportion of respondents who chose particular options.
- Understanding probabilities: Probability is often expressed as a percentage, representing the likelihood of a specific event occurring.
3. Everyday Life:
- Calculating tips: Determining the appropriate tip amount in a restaurant involves calculating a percentage of the bill.
- Understanding sales tax: Sales tax is a percentage of the purchase price.
- Measuring progress: Tracking progress towards goals, such as completing a project or achieving a fitness target, can be conveniently represented using percentages.
Further Exploring Percentage Calculations:
Beyond the simple calculation of "What percentage is 4 out of 24?", let's explore some related concepts that will enhance your understanding of percentages:
Finding the Percentage Increase or Decrease
Calculating the percentage increase or decrease is useful in various situations, such as comparing sales figures or tracking changes in population. The formula is:
[(New Value - Old Value) / Old Value] x 100%
For instance, if sales increased from 100 to 120, the percentage increase would be:
[(120 - 100) / 100] x 100% = 20%
Calculating a Percentage of a Number
To find a certain percentage of a number, convert the percentage to a decimal and multiply by the number. For example, to find 15% of 200:
0.15 x 200 = 30
Finding the Original Value After a Percentage Change
If you know the final value after a percentage increase or decrease, you can work backward to find the original value. This often involves solving an equation.
For example, if a price increased by 10% to reach $110, the original price (x) can be found by solving:
x + 0.1x = 110 1.1x = 110 x = 100
The original price was $100.
Tips and Tricks for Mastering Percentage Calculations
- Practice regularly: The more you practice, the more confident and proficient you'll become.
- Use different methods: Try all the methods discussed above to find the one that suits you best.
- Understand the concepts: Don't just memorize formulas; grasp the underlying principles of percentages.
- Use online resources: There are many online calculators and tutorials available to help you learn and practice.
- Check your work: Always double-check your calculations to ensure accuracy.
Conclusion: Mastering the Art of Percentages
Understanding how to calculate percentages, and specifically answering the question "What percentage is 4 out of 24?", is a vital skill applicable to numerous aspects of life. By mastering the techniques and understanding the concepts discussed in this guide, you'll be well-equipped to confidently handle percentage calculations in any context. Remember that consistent practice and a solid grasp of the underlying principles are key to achieving proficiency. So, start practicing, and you'll soon find yourself effortlessly navigating the world of percentages!
Latest Posts
Latest Posts
-
What Is Half Of A Centimeter
May 11, 2025
-
How Many Minutes Are In 9 Days
May 11, 2025
-
Del 2007 Al 2024 Cuantos Anos Son
May 11, 2025
-
What Is 5 10 As A Percentage
May 11, 2025
-
How Much Is 1 5 Fluid Ounces
May 11, 2025
Related Post
Thank you for visiting our website which covers about What Percentage Is 4 Out Of 24 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.