8 Out Of 30 Is What Percent
Treneri
May 13, 2025 · 4 min read
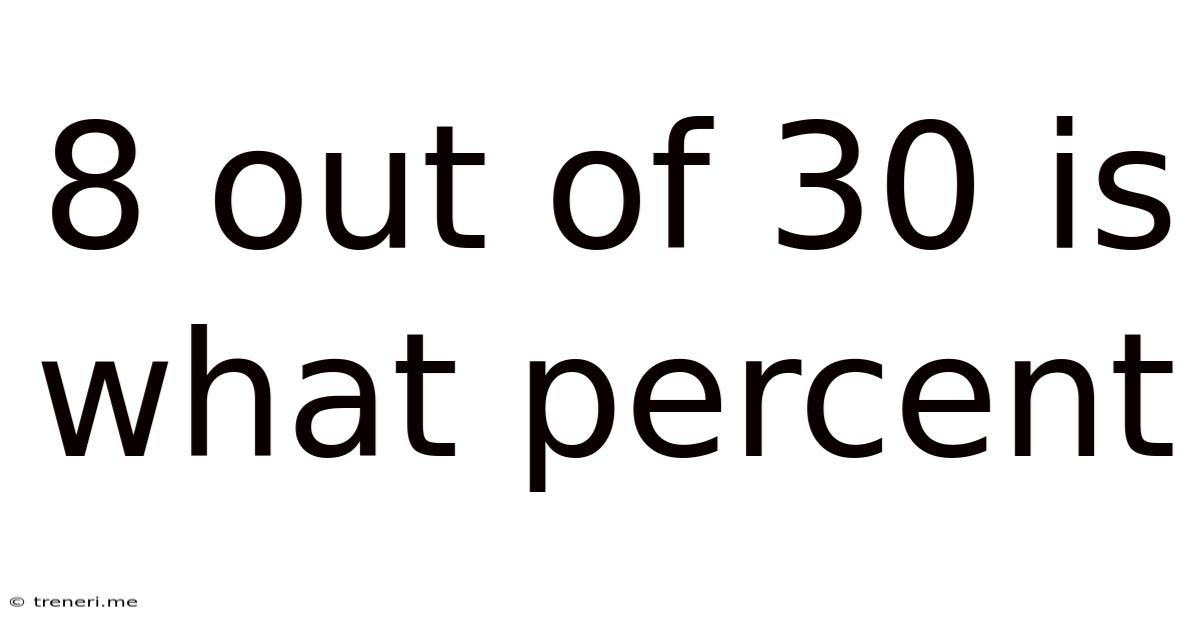
Table of Contents
8 Out of 30 is What Percent? A Comprehensive Guide to Percentage Calculations
Understanding percentages is a fundamental skill applicable across various aspects of life, from calculating discounts and tips to analyzing data and understanding statistics. This comprehensive guide will delve into how to calculate what percentage 8 out of 30 represents, and more importantly, equip you with the knowledge to solve similar problems independently. We'll explore different methods, discuss the underlying concepts, and provide real-world examples to solidify your understanding.
Understanding Percentages: The Basics
Before diving into the calculation, let's solidify our understanding of percentages. A percentage is a fraction or ratio expressed as a number out of 100. The symbol "%" represents "per cent," meaning "out of one hundred." Therefore, 50% means 50 out of 100, which is equivalent to ½ or 0.5 in decimal form.
Method 1: Using the Formula
The most straightforward method for calculating percentages involves a simple formula:
(Part / Whole) * 100% = Percentage
In our case:
- Part: 8
- Whole: 30
Applying the formula:
(8 / 30) * 100% = 26.67% (approximately)
Therefore, 8 out of 30 is approximately 26.67%.
Method 2: Converting to a Decimal
This method involves converting the fraction to a decimal and then multiplying by 100%.
- Convert the fraction: 8/30 = 0.2667 (approximately)
- Multiply by 100%: 0.2667 * 100% = 26.67% (approximately)
This method yields the same result as Method 1, confirming our answer.
Method 3: Using Proportions
Proportions offer a visual and intuitive approach to solving percentage problems. We can set up a proportion:
8/30 = x/100
Where 'x' represents the percentage we want to find. To solve for 'x', we cross-multiply:
30x = 800
x = 800 / 30
x ≈ 26.67
This method again confirms our answer.
Understanding the Approximation
It's important to note that 26.67% is an approximation. The exact decimal representation of 8/30 is a repeating decimal (0.266666...). We typically round to two decimal places for practical purposes. The level of precision required depends on the context of the problem. In some cases, rounding to the nearest whole number (27%) might be sufficient.
Real-World Applications
Understanding percentage calculations has numerous practical applications. Let's explore a few scenarios:
Scenario 1: Test Scores
Imagine a student scored 8 out of 30 on a quiz. Using our calculation, we know they achieved a score of approximately 26.67%. This information helps assess their performance relative to the total possible marks.
Scenario 2: Sales and Discounts
A store offers a discount on 8 out of 30 items. This represents a 26.67% discount on the selection of items. This percentage helps customers quickly understand the extent of the sale.
Scenario 3: Data Analysis
In data analysis, percentages are crucial for interpreting results. For example, if a survey shows 8 out of 30 respondents prefer a particular product, it indicates that approximately 26.67% of respondents have this preference. This percentage provides a clear representation of the data.
Scenario 4: Financial Calculations
Percentages are fundamental in financial calculations. Interest rates, returns on investment, and tax rates are all expressed as percentages. Understanding percentage calculations is essential for managing personal finances and making informed financial decisions.
Advanced Percentage Calculations: Beyond the Basics
While calculating 8 out of 30 as a percentage is a straightforward example, understanding percentages extends to more complex calculations. Let's explore some of them:
Calculating Percentage Increase or Decrease
Suppose a value increases from 30 to 38. To calculate the percentage increase:
- Find the difference: 38 - 30 = 8
- Divide the difference by the original value: 8 / 30 = 0.2667
- Multiply by 100%: 0.2667 * 100% = 26.67%
Therefore, there's a 26.67% increase. A similar method is used for calculating percentage decreases.
Finding the Original Value
If you know the percentage and the final value, you can calculate the original value using reverse percentage calculations. For instance, if a value increased by 26.67% to become 38, we can find the original value as follows:
Let x be the original value. x + 0.2667x = 38 1.2667x = 38 x = 38 / 1.2667 x ≈ 30
This confirms our original value was 30.
Working with Multiple Percentages
Often you’ll encounter situations involving multiple percentages. For example, calculating a discount after a discount, or compound interest. These require careful attention to the order of operations and often involve iteratively applying the percentage calculations.
Practical Tips and Troubleshooting
- Double-check your calculations: Always verify your calculations to avoid errors.
- Use a calculator: Calculators significantly ease the process, especially for more complex calculations.
- Understand the context: The level of precision needed depends on the context. Rounding appropriately is crucial.
- Practice regularly: Consistent practice strengthens your understanding and improves your speed and accuracy.
- Seek help when needed: Don't hesitate to seek help if you encounter difficulties. Numerous online resources and tutorials are available.
Conclusion
Calculating what percentage 8 out of 30 represents—approximately 26.67%—is a fundamental skill in various fields. Understanding the underlying principles and various calculation methods is crucial for successfully tackling percentage problems of varying complexity. By mastering percentage calculations, you significantly enhance your ability to interpret data, solve practical problems, and make informed decisions in various aspects of life. Remember to practice regularly and utilize available resources to solidify your understanding and become proficient in this essential mathematical skill. This guide provides a solid foundation; continuous learning and application will further strengthen your expertise in this area.
Latest Posts
Latest Posts
-
What Is 2 To The Power Of 9
May 13, 2025
-
2 7 8 Inches Ring Size
May 13, 2025
-
How Is Margin Of Safety Calculated
May 13, 2025
-
What Fractions Are Equivalent To 3 9
May 13, 2025
-
19 As A Fraction Of An Inch
May 13, 2025
Related Post
Thank you for visiting our website which covers about 8 Out Of 30 Is What Percent . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.