What Percentage Is 78 Of 120
Treneri
May 13, 2025 · 4 min read
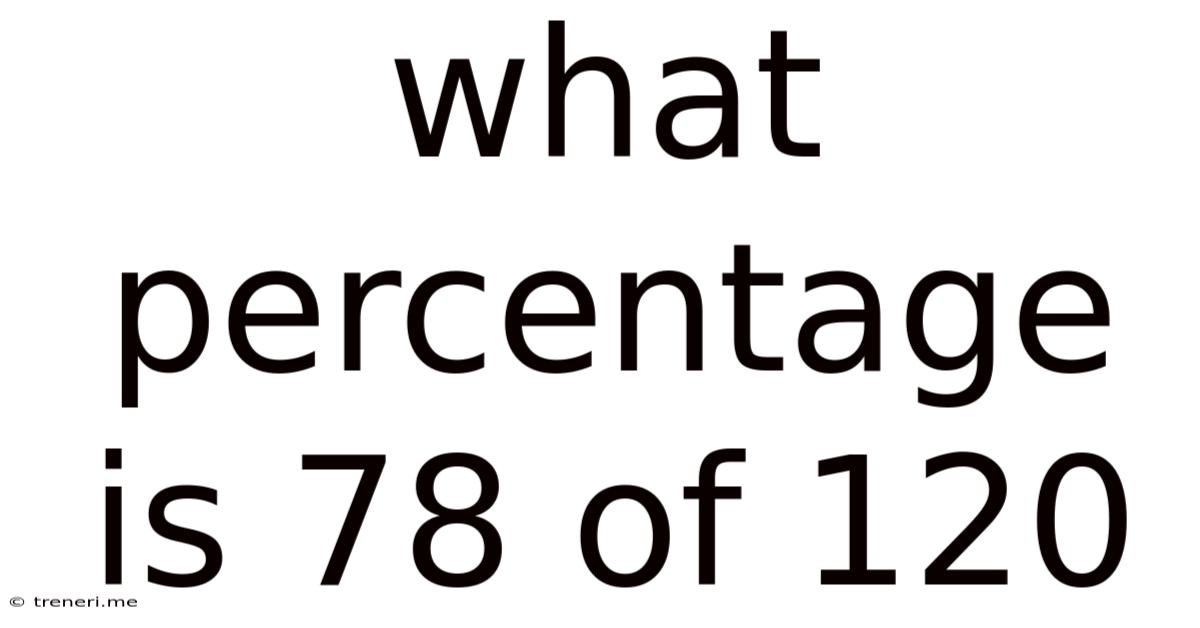
Table of Contents
What Percentage is 78 of 120? A Comprehensive Guide to Percentage Calculations
Understanding percentages is a fundamental skill applicable across numerous fields, from finance and statistics to everyday life. This article delves into calculating what percentage 78 represents of 120, explaining the process in detail and exploring various methods. We'll also discuss practical applications and how to perform similar calculations efficiently.
Understanding Percentages
A percentage is a way of expressing a number as a fraction of 100. The term "percent" literally means "out of one hundred" (per centum in Latin). Percentages are represented using the "%" symbol. For instance, 50% means 50 out of 100, or 50/100, which simplifies to 1/2 or 0.5.
Calculating the Percentage: 78 of 120
To determine what percentage 78 represents of 120, we follow these steps:
Step 1: Set up the Proportion
We can express this problem as a proportion:
78/120 = x/100
Where 'x' represents the percentage we want to find.
Step 2: Solve for x
To solve for 'x', we cross-multiply:
78 * 100 = 120 * x
7800 = 120x
Step 3: Isolate x
Divide both sides of the equation by 120:
x = 7800 / 120
x = 65
Therefore, 78 is 65% of 120.
Alternative Methods for Percentage Calculation
While the proportion method is straightforward, several other approaches can be used to calculate percentages:
Method 1: Using Decimal Conversion
- Divide the part by the whole: 78 / 120 = 0.65
- Multiply by 100 to convert to a percentage: 0.65 * 100 = 65%
This method directly converts the fraction to a decimal and then to a percentage.
Method 2: Using a Calculator
Most calculators have a percentage function. Simply input "78 ÷ 120 * 100 =" to get the result: 65%.
Method 3: Simplifying the Fraction
Before converting to a percentage, you can simplify the fraction 78/120. Both numbers are divisible by 6:
78/120 = 13/20
Now, convert the simplified fraction to a percentage:
(13/20) * 100 = 65%
This method simplifies the calculation, especially when dealing with larger numbers.
Practical Applications of Percentage Calculations
Understanding percentage calculations has wide-ranging applications:
1. Finance:
- Interest rates: Calculating interest earned on savings accounts or paid on loans.
- Discounts: Determining the final price after a percentage discount is applied.
- Taxes: Calculating sales tax or income tax.
- Investment returns: Assessing the profitability of investments.
2. Statistics:
- Data analysis: Representing data as percentages to illustrate proportions and trends.
- Probability: Expressing the likelihood of events occurring as percentages.
- Surveys and polls: Presenting results as percentages to show public opinion.
3. Everyday Life:
- Tip calculations: Determining the appropriate tip amount in a restaurant.
- Sales and discounts: Comparing prices and finding the best deals.
- Recipe scaling: Adjusting ingredient quantities based on percentage changes.
- Grade calculations: Determining your final grade in a course.
Advanced Percentage Problems and Solutions
Let's explore some more complex percentage problems and how to solve them.
Problem 1: Finding the Whole when given a Percentage and Part
-
Question: 65% of a number is 78. What is the number?
-
Solution:
Let the number be 'y'. We can set up the equation:
0.65 * y = 78
y = 78 / 0.65
y = 120
Therefore, the number is 120.
Problem 2: Finding the Percentage Increase or Decrease
-
Question: A product's price increased from $100 to $120. What is the percentage increase?
-
Solution:
- Find the difference: 120 - 100 = 20
- Divide the difference by the original price: 20 / 100 = 0.2
- Multiply by 100 to convert to a percentage: 0.2 * 100 = 20%
The price increased by 20%.
Problem 3: Calculating Percentage Points
Percentage points represent the arithmetic difference between two percentages. It's crucial to distinguish between a percentage change and percentage points.
- Example: If interest rates increase from 5% to 8%, the increase is 3 percentage points, not 60% (which would be the percentage change).
Tips for Accurate Percentage Calculations
- Double-check your work: Always verify your calculations to minimize errors.
- Use a calculator: For complex calculations, a calculator can save time and improve accuracy.
- Understand the context: Pay attention to the wording of the problem to ensure you're performing the correct calculation.
- Practice regularly: The more you practice, the more comfortable and proficient you'll become with percentage calculations.
Conclusion
Calculating percentages is a vital skill with wide-ranging applications. Understanding the different methods, from setting up proportions to using decimal conversions, allows you to approach various percentage problems efficiently. By mastering these techniques, you can confidently tackle numerous situations requiring percentage calculations in your personal and professional life. Remember to always double-check your work and practice regularly to improve your accuracy and speed. The ability to work with percentages accurately is a key element of numerical literacy and essential for success in various fields.
Latest Posts
Latest Posts
-
2 3 Times What Equals 1
May 13, 2025
-
How Many Miles Is 750 Km
May 13, 2025
-
How Many Cups Is Six Quarts
May 13, 2025
-
What Is 2 1 As A Fraction
May 13, 2025
-
How Many Pounds Is 7 Stones
May 13, 2025
Related Post
Thank you for visiting our website which covers about What Percentage Is 78 Of 120 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.